Question
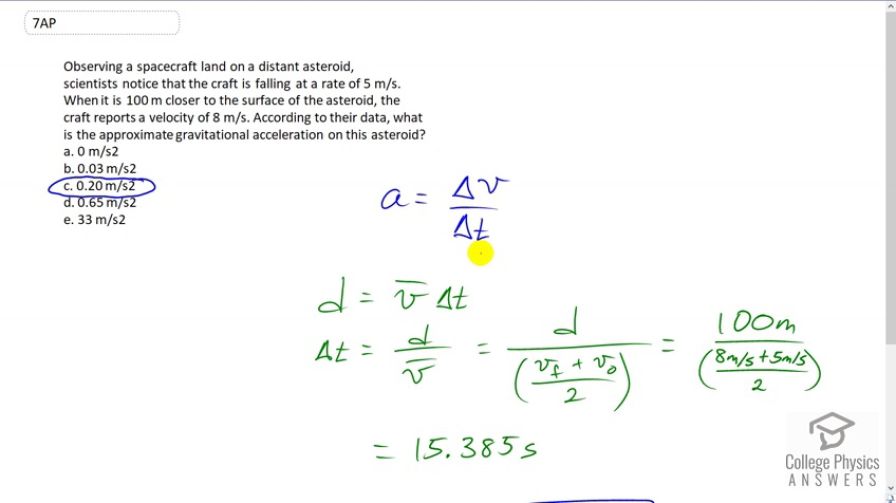
Observing a spacecraft land on a distant asteroid, scientists notice that the craft is falling at a rate of 5 m/s. When it is 100 m closer to the surface of the asteroid, the craft reports a velocity of 8 m/s. According to their data, what is the approximate gravitational acceleration on this asteroid?
-
0 m/s2
-
0.03 m/s2
-
0.20 m/s2
-
0.65 m/s2
-
33 m/s2
Final Answer
(c)
Solution video
OpenStax College Physics for AP® Courses, Chapter 2, Problem 7 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We can find the acceleration of this spacecraft by dividing its change in velocity by the change it time over which that velocity change occurs. Now, we're not given the change in time though, so that will make this question a bit tricky. We are given the distance that the spacecraft covers between a speed of 5 meters per second and 8 meters per second, and so we have to use this formula that the distance equals the average velocity multiplied by the change in time, in order to find out what the change in time is. And then we'll plug that back into our acceleration formula to get our answer. And so, we rearrange this formula here for displacement by dividing both sides by v bar the average velocity, and switch the sides around. And we get delta t is d over v bar and v bar is the arithmetic average of the final and initial velocities, so that's v f plus v i over 2. That's 100 meters that it gets closer to the asteroid, divided by final velocity of 8 meters per second plus the initial velocity of 5, all divided by 2. That gives 15.385 seconds. So that's our denominator in the acceleration formula and so we have 8 meters per second minus 5, the change in velocity divided by the time gives us about 0.2 meters per second squared which is option C.
