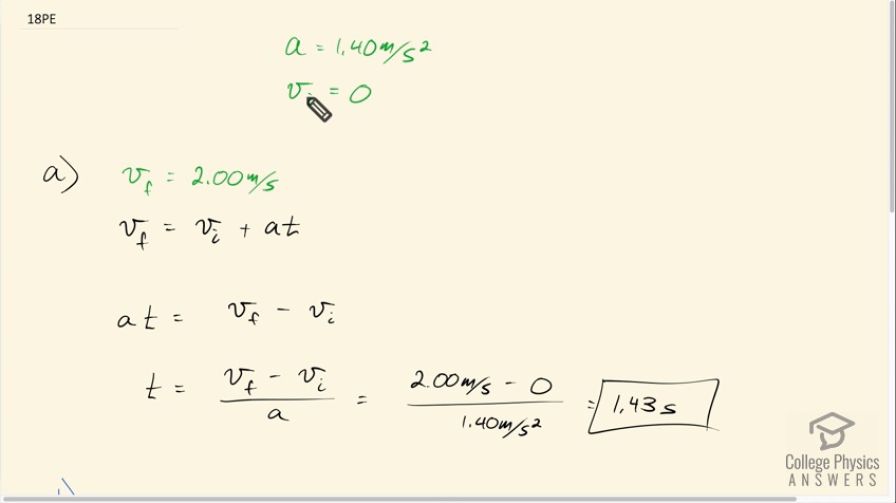
Question
A commuter backs her car out of her garage with an acceleration of . (a) How long does it take her to reach a speed of ? (b) If she then brakes to a stop in , what is her deceleration?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 2, Problem 18 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This commuter backed out of the driveway with an acceleration of 1.40 meters per second squared; we can assume that their initial velocity is 0 since they are starting in their driveway at rest. The final velocity for part (a) we are told is 2.00 meters per second and the question is how long does it take or to get from 0 to 2 meters per second? So final velocity is initial velocity plus acceleration times time and we can rearrange this for at by subtracting v i from both sides and then switch the sides around and we get acceleration times time equals final velocity minus initial velocity and then solve for t by dividing both sides by a and so we get time is the difference in velocity divided by acceleration. So that's 2 meters per second—final velocity— minus 0—initial velocity— divided by 1.40 meters per second squared—acceleration— giving a time of 1.43 seconds. In part (b), we are told that they brake and then eventually stop so they have a final velocity of 0 in 0.8 seconds and the initial velocity we assume is what they had in part (a), 2 meters per second; this is the final velocity of part (a). So we are gonna rearrange this to solve for acceleration and we start with the same formula here, v f equals v i plus at and then subtract v i from both sides as we did before and then we have at equals v f minus v i and this time instead of dividing both sides by a, we are gonna divide both sides by t to solve for a. So acceleration is final velocity minus initial velocity divided by time so that's 0 minus 2 meters per second divided by 0.800 seconds which is negative 2.50 meters per second squared and the negative sign indicates that change of velocity is in the negative direction or if you start with a positive velocity—as we do— a negative acceleration will slow it down.
