OpenStax College Physics for AP® Courses, Chapter 2, Problem 15 (Problems & Exercises)

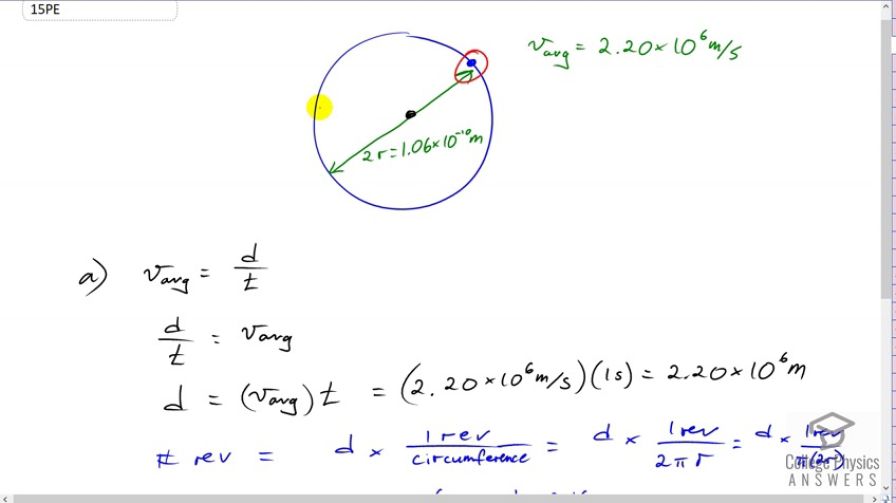
This is College Physics Answers with Shaun Dychko In this solution, we're going to calculate the number of revolutions that this electron makes in the hydrogen atom around the nucleus in a second. Now, we're told that the diameter of its circular path is 1.06 times 10 to the minus 10 meters, and I've written 2r here instead of diameter just to reduce confusion with the variable letters that I've chosen, because down here when I talk about average speed, I'm saying it's distance traveled over time and I don't want that d to be confused with diameter. So, I wrote 2r over there. We're told that the average speed is 2.2 times 10 to the 6 meters per second. So, notice what I've done at the beginning here, is I've drawn a picture of the situation, and I have written down the information that we've been given. That's a really good way to start every problem really because that helps organize your thoughts and organize the information you begin and then you can find a solution easier that way. So, we're going to figure out what the total distance traveled is first and that'll give us an answer in meters. And then, knowing the size of the circle, we'll figure out how many revolutions make up that many meters. So, we'll find revolutions basically by converting the distance traveled from meters into revolutions by multiplying by one revolution for every so many meters circumference of the circle. So, we switch the two sides around here because we want to isolate d in the left and where you will multiply both sides by t in this line, and the ts cancel on the left making d by itself there. And so, d equals average speed multiplied by time. So, that's 2.2 times 10 to the 6 meters per second times 1 second and that makes 2.2 instead of 6 meters. And then, we'll find the number of revolutions in that distance traveled by multiplying the distance traveled by one revolution for every circumference of the circle, which is 2 Pi r, r being the radius. And... And up here, we have a number representing 2r. And so, let's move these factors around a little bit and just group the 2 beside the r. And then, we can substitute the number we've been given in the question directly for 2r, which by the way is diameter D in the formula for circumference of... Circumference is Pi D by the way. So, we substitute for 2r. We substitute 1.06 times 10 to the minus 10 meters and multiply that by pi in the denominator. All that gets divided into 2.2 times 10 to the 6 meters and we are left with 6.61 times 10 to the 15 revolutions of the electron around the nucleus per second. Now for part B, it says, 'What's the average velocity?' and they like to be tricky with these velocity questions because the electron after one complete circle returns to its starting position. Its displacement is 0 and so the velocity is 0.