OpenStax College Physics for AP® Courses, Chapter 2, Problem 58 (Problems & Exercises)

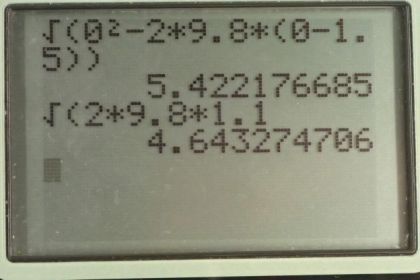
This is College Physics Answers with Shaun Dychko. A ball is dropped from a height of 1.5 meters and it achieves some maximum velocity which we'll call v 1— just before it hits the ground here— and we'll figure out what that velocity is in part (a). And then it rebounds with a velocity which we'll call v 1 prime keeping the subscript 1 because it's a velocity at the same position here but this velocity is different because the bounce is not perfectly efficient and so some energy is lost and so this rebound velocity, v 1 prime, is going to be less than v 1 was when it was going downwards. And then it will reach some maximum height of 1.10 meters which we'll call position 2 and it will have some velocity 2 of zero there as everything does when it reaches a maximum height, velocity is always zero at the very maximum of an arc or of something just going through the air. So we find v 1 going downwards here in part (a); in part (b), we find v 1 prime, the rebound velocity; in part (c), we find the acceleration of this tennis ball when it's in contact with the ground and then in part (d), we figure out how much the tennis ball is compressed. So it starts with some spherical diameter here and gets compressed into this ellipse and we'll figure out how much that compression is. Okay. So we are going to figure out what is velocity v 1 then and we know that v 1 squared equals v initial squared minus 2 times acceleration due to gravity multiplied by the final height minus the initial height—that's equation 77— and we are gonna solve for v 1 by taking the square root of both sides and that is the plus or negative square root and we have to use our knowledge of the physical situation to decide whether it should be positive or negative. Now because the tennis ball is moving down and we usually take upwards to be the positive direction that means this velocity is going to be negative. So we choose the negative here and we have the negative square root of 0 meters per second squared— that's v naught is zero, it was dropped and so whenever the question says the word 'dropped' that means we are given no initial velocity— minus 2 times acceleration due to gravity, 9.80 meters per second squared, multiplied by the final height which is 0 meters at the ground level, y 1, minus the initial height of 1.50 meters and this works out to negative 5.42 meters per second. In part (b), we want to know the rebound velocity, v 1 prime, and we know that v 2 squared, the velocity at the very top of its rebound when it's at a height of 1.10 meters, v 2 squared equals the initial velocity, v 1 prime squared, minus 2g times the final height of y 2 minus the initial height of y 1 so it gets to this final height, y 2, and it started down here at y 1. And we are gonna solve for v 1 prime by adding 2g y 2 minus y 1 to both sides and this v 2 is 0 so we are just gonna make that term disappear and switching the sides around, we have v 1 prime on the left here and we have also taken the square root of both sides and so that's why we have a square root of 2g times y 2 minus y 1 here. And we'll take the positive now because we can see that the rebound is going upwards so v 1 prime is positive. So we have positive square root of 2 times acceleration due to gravity multiplied by the final height of 1.10 meters minus the initial height at the ground of 0 meters giving us a velocity, v 1 prime, of 4.64 meters per second. The acceleration of the tennis ball, while it's in contact with the ground is the final velocity, which is the rebound velocity, minus the initial downward velocity just before it contacted the ground divided by the time it spends in contact with the ground which we are told is 3.5 milliseconds. So we have 4.6433 meters per second— rebound velocity— minus the negative— and it's important to notice that there's a minus from the formula and then a negative for the velocity v 1 together is gonna make a positive— 5.4222 meters per second divided by 3.50 milliseconds written as times 10 to the minus 3 seconds giving us 2.88 times 10 to the 3 meters per second squared and that's a positive acceleration. Okay. So in part (d), we are going to figure out how much the tennis ball is compressed while it's in contact with the ground. So we consider this initial moment when it just begins to contact the ground and at this point, we are gonna consider this very top point of the ball and we'll follow it through to the point where the ball is fully compressed and at this point, when the ball is compressed, this point at the top of the ball will have zero velocity whereas initially, it has a velocity of negative 5.4222 meters per second and I'm calling this v naught, which is sort of a relabelling of what I called v 1 before but let's reset our subscripts here and call this v naught so that we can write our formulas the way we are used to seeing them. Okay. So this point has a velocity v naught downwards and it has an acceleration upwards due to being in contact with the ground and that acceleration is what we calculated in part (c). And then despite the acceleration upwards, it is nevertheless still going down because the acceleration has not been applied for long enough to reverse the direction of this point and so it's continuing down despite an acceleration upwards but it's slowing down very rapidly to the point where it finally gets to a velocity of zero and we want to know what is the difference between these two positions of the top of the ball; that will be our compression, that is this initial height of y naught minus the final height of y. So we have equation 54 which tells us that the final velocity squared equals the initial velocity squared plus 2 times acceleration times the final position minus the initial position and we are gonna solve for y minus y naught and we'll do that by subtracting v naught squared from both sides and then also noticing this term is zero because the final velocity is zero and we have, after switching the sides around, we have 2a times y minus y naught equals negative v naught squared. Then we are gonna divide both sides by negative 2a and so these negative make a positive so that's positive v naught squared over 2a and on the left side, this negative is gonna switch the signs of these two terms around so that we have y naught minus y and that's a bit nicer to write it this way because we know that that's going to give us a positive number and it's just nice to express our compression as a positive amount, a magnitude. So we have y naught minus y equals v naught squared over 2a so that's negative 5.4222 meters per second squared divided by 2 times the acceleration that we calculated in part (c) and that gives 0.0051116 meters which is 5.11 millimeters. So it compresses about half a centimeter while it's in contact with the ground.