Question
There is a 250-m-high cliff at Half Dome in Yosemite National Park in California. Suppose a boulder breaks loose from the top of this cliff. (a) How fast will it be going when it strikes the ground? (b) Assuming a reaction time of 0.300 s, how long will a tourist at the bottom have to get out of the way after hearing the sound of the rock breaking loose (neglecting the height of the tourist, which would become negligible anyway if hit)? The speed of sound is 335 m/s on this day.
Final Answer
a)
b)
Solution video
OpenStax College Physics for AP® Courses, Chapter 2, Problem 53 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
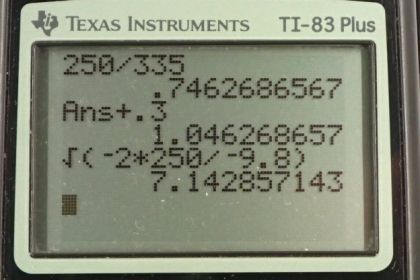
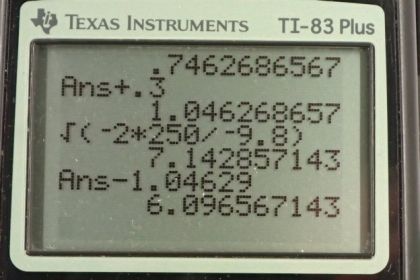
Calculator Screenshots
Video Transcript
This is College Physic Answers with Shaun Dychko. Suppose a boulder falls from the top of this half dome in Yosemite National Park and that top of the half dome is at a position 250 meters above this tourist. We'll say that the tourist's position is y equals zero and I'm going to put a subscript three there by the way which becomes relevant down here. But anyway, so part A simply asks what is the speed of this boulder when it hits the ground. The acceleration will be that due to gravity of negative 9.8 meters per second squared. We assume it has no initial velocity 'cause it's just falling off the cliff, hasn't been thrown down and we can use this formula, final speed squared equals initial velocity squared plus two times acceleration, times the final position minus the initial position. The initial velocity is zero so this term disappears, and so we have v squared is, well v squared will be two a times y minus y naught. Then v, can erase that exponent there, take the square root of both sides, v becomes square of two a times final position minus initial position. So that's the square root of two times negative 9.8 meters per second squared acceleration, times zero the final height, minus the initial height of 250 meters and we get 70 meters per second is the final velocity, or the final speed I should say, of the boulder when it hits the ground. The velocity would be negative and yeah -- the wording of the question says how fast will it be going when it strikes the ground. So that suggests a speed, how fast is it going. It's not asking for the velocity. It's asking for the speed. So this would be the answer, positive 70 meters per second 'cause speeds are always positive. Speed is the magnitude of the velocity. Technically speaking if it was asking for a velocity, you would answer negative 70 meters per second because it's directed down. Okay. Now for part B, I re-drew the drawing up above and pasted it here and we have a few intervals of time to consider. So this boulder when it breaks off of the cliff, it creates some sound and it takes time for that sound to reach the hiker's ears. Then we're going to call that time t one. So the boulder would have fallen to here by the time the sound of it breaking loose has reached the hiker. Then the hiker has some reaction time. After they hear the sound it takes some time for their body to, you know, their mind to process the sound and their body muscles to start moving. We're going to that t subscript capital R for reaction time and t two is the time after the reaction time has finished. So, t two includes the time for the sound to reach the hiker and it also includes the reaction time. So this interval between these two is the reaction time and t two itself is the reaction time plus t one. So, the time that the hiker has to get out of the way then, is is the total time it takes for the rock to hit the ground which is t three, minus t two. So it's this interval of time that the hiker has to get out of the way because there is some time spent before the sound reaches the hiker and then there is some time spent while the hiker is not doing anything, just reacting, reaction time. Then finally, this is the amount of time they have to get out of the way. That'll be t three minus t two. Okay. So first question, what is t one? That is the time for sound emitted at the top of the cliff to reach the hiker. There's no acceleration involved here because it's sound traveling, that's not an object moving. It's energy moving, and so we have the cliff height divided by the speed of sound. So it's 250 meters divided by 335 meters per second which is 0.74627 seconds. Now, t two is that time for sound to reach the hiker plus the hiker's reaction time and we're told that the reaction time is 0.3 seconds. So we add those to get 1.0463 seconds. Okay. Now, the question is what is t three, the time at which the boulder hits the ground. We have that position three equals initial position of the boulder at the top of the cliff, plus its initial velocity which is zero so this term disappears actually, plus one half times acceleration, times t three squared. The final position also is zero, so if zero equals y naught plus one half at squared, we're going to switch the sides around by subtracting y naught from both sides. So that isolates one half at three squared. But actually now, we switch the sides around and so we have the unknown on the left and this negative y naught on the right. Then, multiply both sides by 2 over a and you get the 2 and the a's canceling on the left and you have them appearing on the right. So you have t three squared equals negative 2y naught over a. Then take the square root of both sides and we're going to take the positive square root because negative time doesn't make physical sense here. So we have t three is the square root of negative 2y naught over a. So that's negative 2 times 250 meters initial height of the boulder, divided by negative 9.8 meters per second squared and that give 7.1429 seconds. That's the total time for the boulder to go from the very top of the cliff to the ground. We'll subtract away from that the time for sound to get to the hiker plus the hiker's reaction time and this gives a total of 1.6 seconds for the hiker to get out of the way. Oh sorry, 6.1 seconds I should say. There!