OpenStax College Physics for AP® Courses, Chapter 15, Problem 6 (Test Prep for AP® Courses)

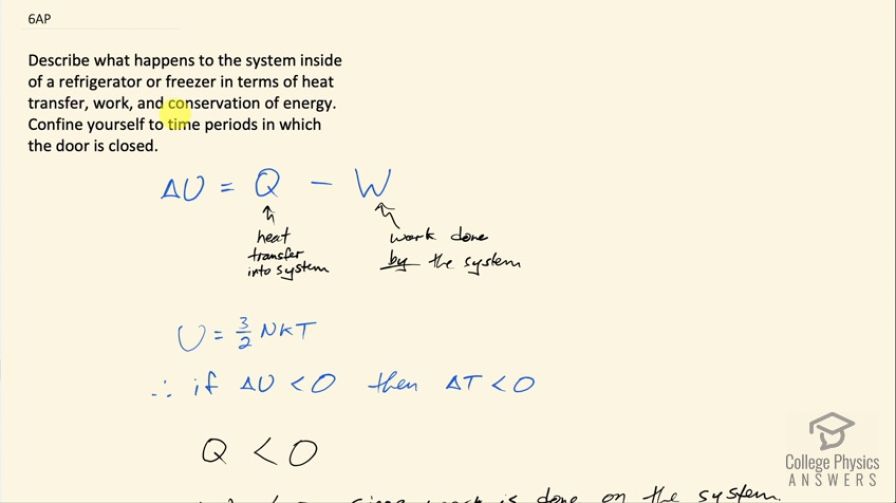
This is College Physics Answers with Shaun Dychko. We are going to describe what happens to the air inside a refrigerator or freezer in terms of heat transfer, work and conservation of energy and we assume that the door is closed on the device. So the change in internal energy, which encompasses both changes in potential and kinetic energy is equal to the heat transfer into a system minus the work done by a system and this change in internal energy is related to temperature because the internal energy equals 3 over 2 times the number of atoms in an ideal monoatomic gas which we are gonna assume that the air approximately is inside the refrigerator multiplied by Boltzmann's constant multiplied by temperature. So if we can show that ΔU is going to be less than zero and in other words, there's a loss in internal energy that will mean that there's a reduction in temperature since temperature is proportional to the internal energy. So for a refrigerator, we know that the amount of heat transferred into the system is negative because in fact heat is taken out of the system— that's the purpose of the device— so Q is going to be less than 0. There's work being done on the system and so that means the work will also be less than zero because this W in the sign conventions of the way this formula is written this W is meant to be the work done by the system but since the work is done on the system in fact, W will be less than 0. Now in order for this refrigerator to be effective, the magnitude of heat removed has to be greater than the work done on the refrigerator so the magnitude of Q has to be greater than the magnitude of W and to use some concrete numbers (I just made up some numbers here) let's suppose that Q is negative 40 joules and then we have minus from our formula and then we have negative amount of work done on the system suppose something of magnitude less than 40 say negative 30 joules in that case, you would have ΔU is negative 10 joules; just to show that with a magnitude of heat greater than the magnitude of work done, we'll end up with a negative answer for our ΔU and since energy is conserved, no energy can spontaneously appear to replace the heat that was removed and so we are left undeniably with this negative change in internal energy, which consequently means there will be a reduction in temperature.