Question
In a very mild winter climate, a heat pump has heat transfer from an environment at to one at .
What is the best possible coefficient of performance for these temperatures? Explicitly show how you follow the steps in the Problem-Solving Strategies for Thermodynamics.
Final Answer
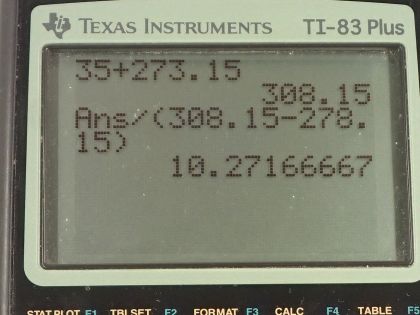
10.3
Solution video
OpenStax College Physics for AP® Courses, Chapter 15, Problem 40 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An ideal heat pump is taking heat out of an environment at 5.00 degrees Celsius and depositing that heat in an environment at 35.0 degrees Celsius and the total amount of heat that it deposits in the high temperature reservoir divided by the work energy it takes to do that transfer is the coefficient of performance. Now we convert each of these temperatures into Kelvin by adding 273.15 and we notice that the Carnot efficiency is the work done divided by the heat that's transferred to the high temperature environment and that's also 1 minus the cold temperature divided by the high temperature and these are both in Kelvin. So this is the reciprocal of this so the coefficient of performance is the reciprocal the efficiency in other words. So we can replace this Q h over w with 1 minus T c over T h to the negative 1 and then we can write this as a single fraction to make it look a bit cleaner by multiplying this by T h over T h and we have T h minus T c over T h to the negative 1 and then we can flip this single fraction and write it as T h over T h minus T c. So the coefficient of performance then is 308.15 Kelvin divided by 308.15 minus 278.15 and that is 10.3.