Question
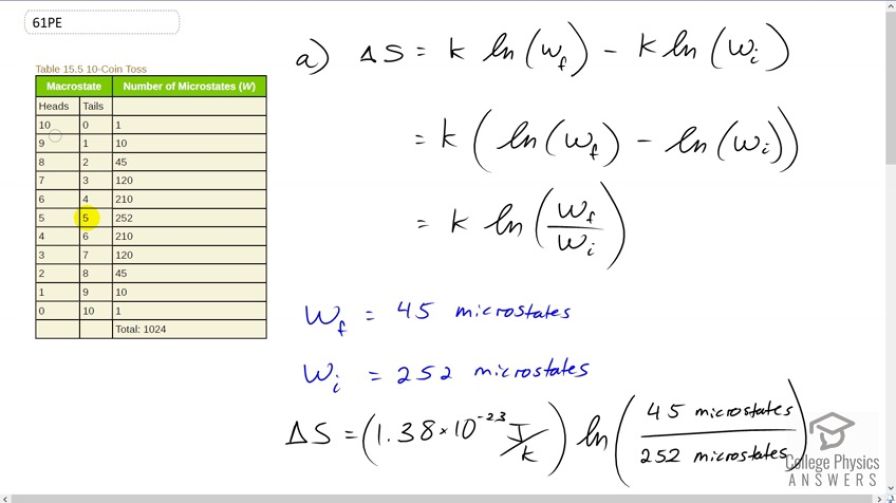
(a) What is the change in entropy if you start with 10 coins in the 5 heads and 5 tails macrostate, toss them, and get 2 heads and 8 tails? (b) How much more likely is 5 heads and 5 tails than 2 heads and 8 tails? (Take the ratio of the number of microstates to find out.) (c) If you were betting on 2 heads and 8 tails would you accept odds of 252 to 45? Explain why or why not.
Final Answer
- 5.6 times as likely
- The \$297 payout is equal to the breakeven payout. One should be ambivalent about accepting these odds.
Solution video
OpenStax College Physics for AP® Courses, Chapter 15, Problem 61 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to find the change in entropy when taking five heads and five tails and then flipping those coins and ending up with two heads and eight tails. So it's going to be an increase in entropy because or should I say decrease in entropy because it's getting from a maximally disordered state of 5 and 5 to a more ordered state of two and eight or it's going from a microstate with many microstates to a microstate of fewer microstates. So we expect change in entropy to be negative. So entropy of a system is Boltzmann constant times the natural logarithm of the number of microstates. And so we have K times natural logarithm of the final number of microstates minus K times natural logarithm of the initial number of microstates and so we factor the K out and we have K times natural logarithm Wf minus natural logarithm Wi. Now when taking the difference of two logarithms we can instead take the logarithm of the quotient of the arguments and you don't have to do this but I just like to do, I like to simplify expressions where I can. So I'm gonna write this as K times natural logarithm of Wf divided by Wi. So the final number microstates is 45 and then the initial number of microstates is 252. So we have the change in entropy then is Boltzmann's constant 1.38 times tenth to the minus 23 joules per Kelvin times natural logarithm of 45 divided by 252 which is negative 2.38 times tenth to the minus 23 joules per Kelvin. So we have a negative reduction in entropy as we expected. Now we wanted to compare the probability of five heads and five tails to the probability of two heads and eight tails. So we divide the number of microstates for each and we get 5.6 . So the five heads and five tails is 5.6 times as likely as two heads and eight tails. Now if you're given a bet with odds of 252 to 45 that means if success occurs and indeed you get two heads and eight tails then you would receive 252 dollars as profit and so the way the betting would work is that you do not give 45 dollars to the bookie instead you keep your money and you say yeah I'll take that bet and then if you win then the bookie gives you 252 dollars. But if you lose then at that point then you have to give the bookie some money and you would give forty five dollars. So in the system you know if you win you get you 252 dollars and you keep your 45 dollars for a total payout of 297 dollars. So the book gives you 252 and you keep 45 and we're gonna call that 297. Now the probability of winning is 45 winning microstates divided by the total number of microstates which is 45 plus 252 because we're taking a bet in this scenario where we started with five and five and flip them and it's gonna be either five and five or two and eight. Not really sure why it has to be only one of those two because there's always other possibilities. But anyway that's the way this question is worded. So there's a probability of a 15 percent of winning each time. So it’s 0.15 wins per trial or per throw of the coins. So the breakeven payout then is suppose you know, this is a bit confusing because the way I'm doing the math assumes that you actually do pay that 45 dollars just to play. So let's look at it that way. So it's 45 dollars per trial and then times one trial for every 0.151515 wins. And this means it's gonna cost 297 dollars per win. Now this breakeven payout is the same as the odds would suggest. And so this is not a good game to play, although you might have some entertainment value in playing in which case you would but you would expect to break even as you play this game many times. And so whether or not to play depends on personal preference and how much you enjoy gambling.

