Question
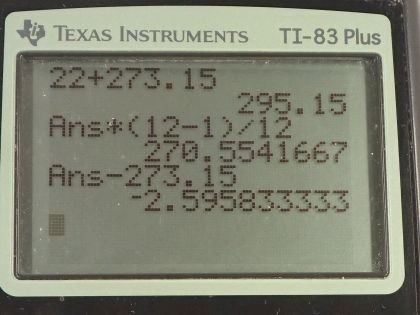
An ideal heat pump is being considered for use in heating an environment with a temperature of . What is the cold reservoir temperature if the pump is to have a coefficient of performance of 12.0?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 15, Problem 44 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Given a heat pump with a coefficient of performance of 12.0 and a high temperature reservoir of 22.0 degrees Celsius, which we convert into Kelvin, what is the temperature of the cold environment from which it is taking heat to deposit into the hot environment? So the coefficient of performance for heat pump is the amount of heat that it delivers to the hot environment divided by the amount of work energy it takes to do that. Now the efficiency is the reciprocal of that— it's the work done divided by the total heat deposited in the hot environment— and for Carnot engine with an ideal efficiency this is also 1 minus the cold temperature divided by the hot temperature. So since this is the reciprocal of the coefficient of performance, we can write the coefficient of performance is the efficiency to the negative 1— the negative 1 being the exponent that flips a fraction and makes it its reciprocal— so this makes the efficiency Q h over w which is what the coefficient of performance is and then we can substitute 1 minus T c over T h in place of efficiency. So 1 minus T c over T h all to the negative 1 is the ideal coefficient of performance for a heat pump but let's write this in a way that's easier to work with because we have to solve for T c. So we multiply this by T h over T h and we get T h minus T c all over T h to the negative 1 and now that we have a single fraction, we can flip it then and so this makes T h over T h minus T c. And then multiply both sides by this denominator T h minus T c... multiply this by T h minus T c and then we end up with this line here so T h minus T c times the coefficient of performance equals T h. So then distribute the coefficient of performance factor into the brackets here we get T h times COP hp minus T c times < i>COP hp equals T h. And then bring this to the right hand side and bring this to the left hand side and also factor it out among these two terms and you get T h times COP hp minus 1 equals T c times COP hp; I have also flipped the sides around as well. Now divide both sides by the COP hp to solve for T c. So the cold temperature environment then is the hot temperature times 1 less than the coefficient of performance divided by the coefficient of performance. So that's 295.15 Kelvin times 12.0 minus 1 divided by 12.0 which is 270.55 Kelvin which after you subtract 273.15 is minus 2.60 degrees Celsius.