OpenStax College Physics, Chapter 15, Problem 25 (Problems & Exercises)

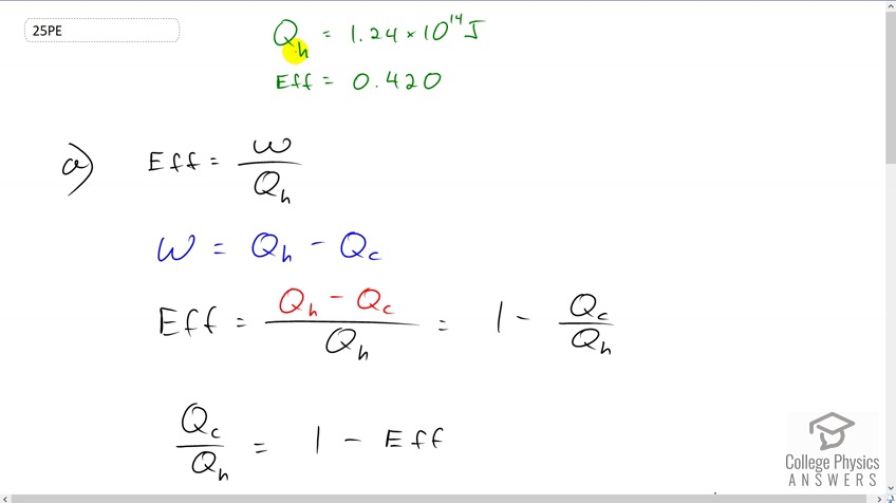
This is College Physics Answers with Shaun Dychko. A power station absorbs 1.24 times 10 to the 14 Joules of energy from the high temperature reservoir and it has an efficiency of 42 percent which is 0.420. In part A, we are asked to figure out how much energy is lost to the cold temperature reservoir so how much energy is just lost to the environment in other words. Well the efficiency is the work done by the power station divided by the energy that it absorbs, and we can rewrite the work as heat energy absorbed minus heat energy lost to the environment and then substitute that in place of W in our efficiency formula. So we can divide both terms in the numerator by this Qh in the denominator and that works out to 1 minus Qc over Qh and we are solving for Qc here and so we will add it to both sides and then subtract efficiency from both sides and we end up with Qc over Qh equals 1 minus efficiency then multiply both sides by Qh to solve for Qc. So Qc equals Qh times 1 minus efficiency. So, it’s 1.25 times 10 to the 14 Joules of energy absorbed multiplied by 1 minus 0.42 efficiency. Giving us 7.25 times 10 to the 13 Joules of energy lost to the environment and then part B asks us to find the ratio of the energy lost to the environment to the amount of the energy produced usefully so the work done, in other words. So, we'll substitute W as Qh minus Qc as we did up here. This is true for any cyclical process that has zero change in internal energy and so we also substitute for Qc and write it as Qh times 1 minus efficiency and now we have an expression containing only efficiency and as well as Qh but that’s gonna end up canceling as we'll see it’s a common factor in the top and bottom. So, we have 1 minus efficiency in the top divided by 1 minus 1 minus efficiency. So, this maybe, an intermediate step would look like this, it would be Qh times 1 minus efficiency divided by Qh times 1 minus 1 minus efficiency. So I’m showing the factoring out of Qh in the denominator there and there's a 1 that appears here. So this bottom 1 minus 1 is 0 and then you know minus minus efficiency makes positive efficiency in the denominator. So we have 1 minus efficiency divided by efficiency in other words. So that’s 1 minus 0.42 divided by 0.42 which is 1.38. So it’s 1.38 times as much energy lost to the environment as there is work done by the power station and the amount of work done is efficiency equals work done divided by Qh and we are gonna solve for work done by multiplying both sides by Qh and so the work done is this 1.25 times 10 to the 14 Joules of energy absorbed times 0.42 which is 5.25 times 10 to the 13 Joules.