Question
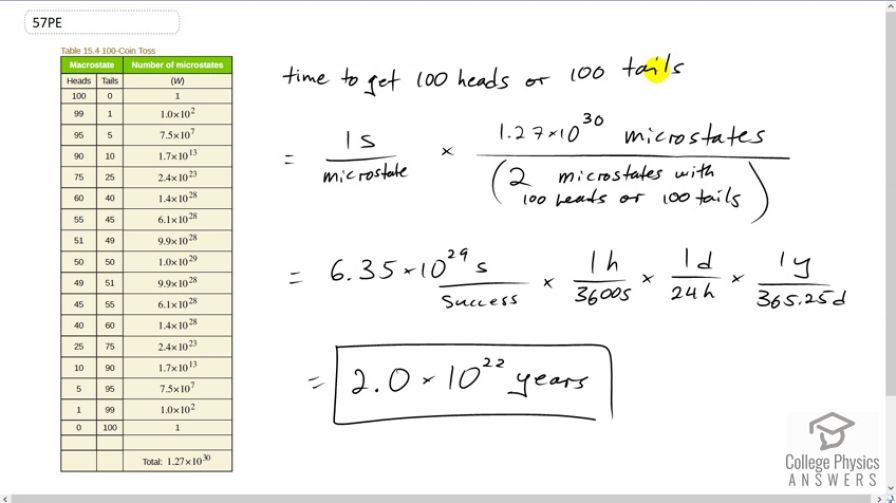
Using Table 15.4, verify the contention that if you toss 100 coins each second, you can expect to get 100 heads or 100 tails once in ; calculate the time to two- digit accuracy.
Final Answer
see the solution video for an explanation.
Solution video
OpenStax College Physics, Chapter 15, Problem 57 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
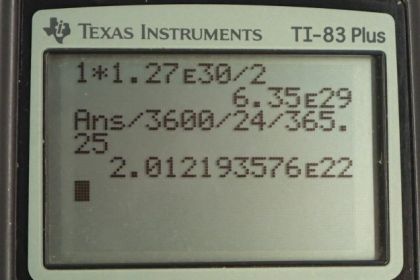
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to calculate the amount of time it would take on average to get 100 heads or 100 tails when flipping 100 coins. So we're flipping at a rate of once per second. So all 100 coins are being simultaneously flipped once every second. And since we're solving for time though we'll take the reciprocal of that and we'll say one second per flip. And I'm using the word microstate here to use the vocabulary from this section. So after you flip the coins one time what you're left with is a collection of heads and tails and that is one microstate. So it's one second per micro state. I mean multiply that by the number of possible microstates. And so these units, micro state units cancel, so timed by 1.27 times tenth to the 30 microstates that's this number here. And then we divide that by the number of microstates that satisfy our condition. So there are two microstates that have 100 heads or 100 tails. So this number two is the total number of success microstates and because there's one microstate for heads and there's one microstate for all tails. And so this works out to 6.35 times tenth to the 29 seconds per success. You might say as success. So there's 1.27 times tenth to the 30 possibilities or microstates divided by two successes and then will convert this into years because we're asked to verify the number of years for this to happen. So we times by one hour for every 3600 seconds times one day per 24 hours times one year for every 365 and a quarter days. And this works out to 2.0 times tenth to the 22 years which is much much longer than the age of the universe.