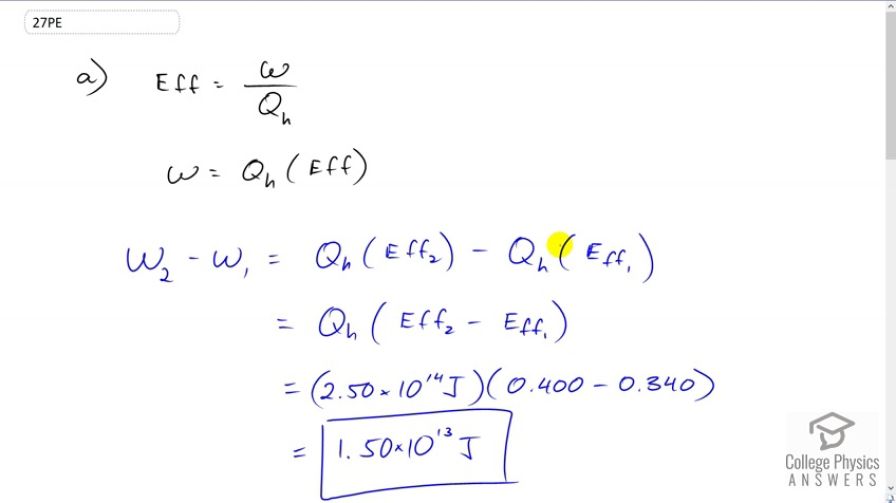
Question
This problem compares the energy output and heat transfer to the environment by two different types of nuclear power stations—one with the normal efficiency of 34.0%, and another with an improved efficiency of 40.0%. Suppose both have the same heat transfer into the engine in one day, . (a) How much more electrical energy is produced by the more efficient power station? (b) How much less heat transfer occurs to the environment by the more efficient power station? (One type of more efficient nuclear power station, the gas-cooled reactor, has not been reliable enough to be economically feasible in spite of its greater efficiency.)
Final Answer
- The more efficient power plant emits less energy to the environment. Notice that this is equal to the additional work done found in part (a).
Solution video
OpenStax College Physics, Chapter 15, Problem 27 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to compare the amount of work done by two different nuclear power plants and we’re given the efficiencies of each and the total amount of energy that each would be absorbing. So efficiency is work done divided by energy absorbed and we can solve for W by multiplying both sides by Qh and so the work done by the more efficient power plant we will call that number two minus the work done by the first power plant. It’s gonna be Qh times efficiency two minus Qh times efficiency one because these are the expressions for work that we just found here from our efficiency formula then we can factor out this common factor Qh from each and so its Qh times the difference in efficiencies is going to be the difference in the works done. So that's 2.5 times 10 to the 14 Joules of energy absorbed times 0.4 minus 0.34 which gives 1.5 times 10 to the 13 Joules of energy of extra energy will be produced in the more efficient nuclear reactor and part B asks us to find the difference in energy lost to the environment so efficiency can be written this way as well, so that's Qh minus Qc all over Qh where I have made a substitution for work done because work done is the difference in energy absorbed and energy lost to the environment for a cyclical process like a power plant or any heat engine and we are going to solve this for Qc. So multiply both sides by Qh and we get Qh minus Qc equals Qh times efficiency, after we switch the sides around and then we'll solve for Qc by moving it to the right and moving this to the left. And we have Qc equals Qh minus Qh times efficiency and then we can factor out the Qh and Qc is Qh times 1 minus efficiency. So the energy lost to the environment for the more efficient nuclear reactor minus that of the less efficient nuclear reactor is going to be Qh times 1 minus efficiency two, minus Qh times 1 minus efficiency one. where I am making substitutions of this for each of the reactors and the Qh can be factored out and then we are left with all this in brackets but this 1 minus efficiency two minus 1 minus efficiency one works out to, efficiency one being positive because there's a negative outside the bracket and the minus inside so that gives two negatives which makes a positive and then minus efficiency two and the ones are subtracted-away to zero. So we have 2.5 times 10 to the 14 Joules of energy absorbed times 0.34 efficiency one minus 0.4 efficiency two which is negative 1.5 times 10 to the 13 Joules and so this negative indicates that the energy lost by the less efficient nuclear reactor is greater and so well that’s what we expected. So the more efficient power plant emits 1.5 times 10 to the 13 Joules less energy to the environment and notice that this is equal to the additional work that is done by the more efficient reactor because that energy has to go somewhere and so it goes into doing work.
