OpenStax College Physics for AP® Courses, Chapter 20, Problem 58 (Problems & Exercises)

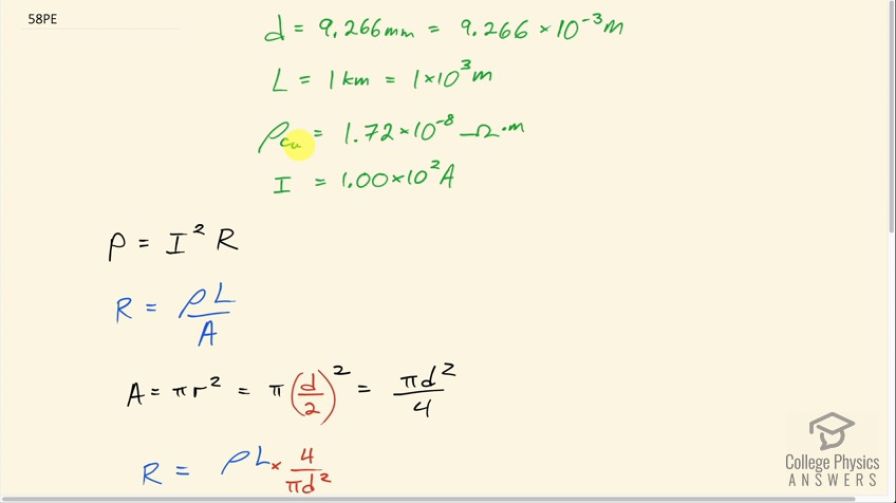
This is College Physics Answers with Shaun Dychko. In this question we want to know how much power is dissipated or lost in a wire that's made out of copper that's 1 kilometer long with a diameter of 9.266 millimeters, when it's carrying a current of 100 amps. So we convert the diameter into meters by multiplying by 10 to the minus 3 since that's what the prefix 'milli' means and then the 'kilo' prefix means multiply by 10 to the 3 and we looked up the resistivity of copper from table [20.1] and it's 1.72 times 10 to the minus 8 ohm-meters and we will need this resistivity to calculate the resistance of this wire because the power it dissipates equals the current that it carries squared multiplied by its resistance and its resistance is the resistivity of the material copper multiplied by its length divided by its cross-sectional area. Now its area will be π times its radius squared and the radius is half the diameter so we substitute d over 2 in place of r and then square that fraction and we get πd squared over 4 is the area and then we will substitute this in for A but instead of dividing a fraction by a fraction, I prefer to multiply by the reciprocal of the denominator so I am gonna flip this over in other words— that's the reciprocal of flipping it over— so I multiplied by 4 over πd squared and all of this can be substituted in place of R in our power formula and so that's why we write power equals current squared times resistivity times length times 4 divided by π times diameter squared. So that's 1.00 times 10 to the 2 amps squared times resistivity of 1.72 times 10 to the minus 8 ohm-meters times 1 times 10 to the 3 meters—length— times 4 divided by π times 9.266 times 10 to the minus 3 meters— diameter—squared and so the power dissipated is 2.55 times 10 to the 3 watts.