Question
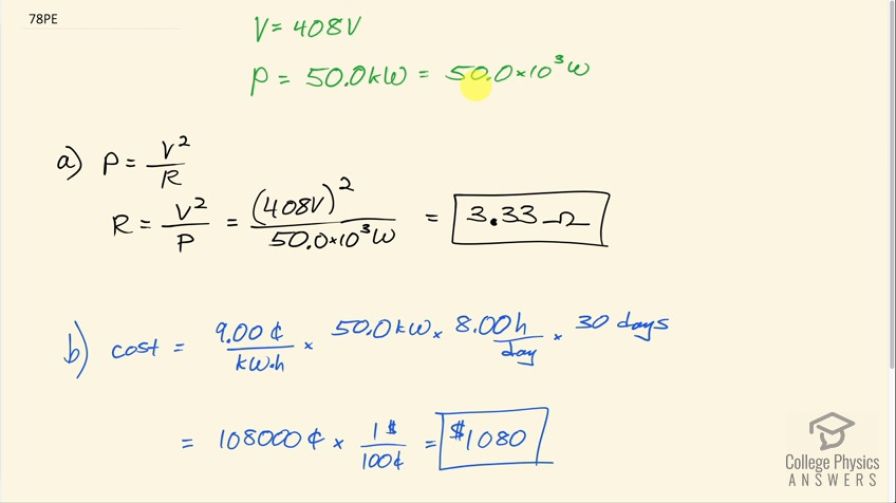
A small office-building air conditioner operates on 408-V AC and consumes 50.0 kW. (a) What is its effective resistance? (b) What is the cost of running the air conditioner during a hot summer month when it is on 8.00 h per day for 30 days and electricity costs ?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 20, Problem 78 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This building's air conditioner runs with a voltage of 408 volts with a power of 50.0 kilowatts, which is 50.0 times 10 to the 3 watts and part (a) asks us what is the resistance effectively of this air conditioner? Well the power is the voltage squared divided by resistance so we can solve for R by multiplying both sides by R over P so the resistance then is the voltage squared divided by the power. So that's 408 volts squared divided by 50.0 times 10 to the 3 watts which is 3.33 ohms. Part (b) asks what is the cost of operating this air conditioner if it runs for 8.00 hours per day for 30 days during the summer months and costs 9.00 cents per kW·h of electricity? So we multiply this electricity rate by the number of kilowatt hours... well so first of all, multiply by the number of kilowatts which is 50.0 and then multiply it by the number of hours that it's running, which is 8.00 hours per day and then by 30 days and this works out to 108000 cents which we convert into dollars by multiplying by 1 dollar for every 100 cents and we are left with $1080.

