OpenStax College Physics for AP® Courses, Chapter 20, Problem 57 (Problems & Exercises)

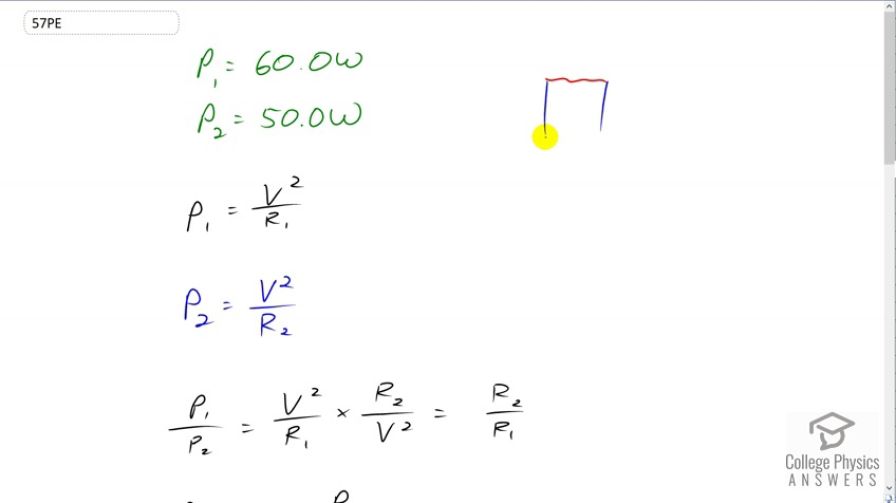
This is College Physics Answers with Shaun Dychko. So there is a light bulb with a filament between the two posts that connect to the electrical supply. This filament has been used for a long time and over time because it's so hot, it has evaporated some of the material that composes the filament and it now has a smaller diameter. So with a smaller diameter it has a greater resistance and so the power that can go through it is going to be reduced. So the power is now 50 watts whereas initially it was 60 watts and so the question is by what ratio has the -- what is the ratio of the diameter now compared to before? Wel,l the power initially would have been the voltage squared divided by its initial resistance. So we have subscripts one to denote the initial factors and then afterwards P two is going to be the same voltage so it does not need a subscript because these will be connected to the same power supply in either case, so the same V squared, but now divided by resistance two. So when we have P one over P two, it's going to be voltage squared over R one, that's P one. Then dividing by this fraction, I prefer instead to multiply by its reciprocal, so multiplied by R two over V squared. So the V squareds cancel leaving us with R two over R one. So R two over R one is P one over P two. Now, our resistance is the resistivty of the material multiplied by its length, divided by its cross-sectional area and I don't need a subscript on the l because the length of this filament is not going to change, only its cross-sectional area will change. So we have rho times l divided by pi times the diameter squared divided by four, this is the cross-sectional area, and then to make it look nicer we'll multiply top and bottom by four to make four rho l over pi d two squared. R one, it's the same idea, is just with diameter one squared. So R two over R one is what we're interested in here. So we have R two is four rho l over pi d two squared and then dividing by R one, we will multiply by its reciprocal. So multiply by pi d one squared over four rho l and that equals P one over P two. A whole bunch of things cancel here. The four and the resistivity, and the l and pi all cancel. We're left with d one squared over d two squared equals P one over P two. Or we could say d two squared over d one squared is P two over P one because we're trying to find the factor by which the new diameter compares to the old diameter so we want d two on top. So d two squared is d one squared times P two over P one. Then take the square root of both sides. So d two is d one multiplied by the square root of >i>P two over P one. So d two is d one times square root of 50 watts over 60 watts and that is 0.913 d one. So the diameter is reduced by a factor of 0.913.