OpenStax College Physics for AP® Courses, Chapter 20, Problem 82 (Problems & Exercises)

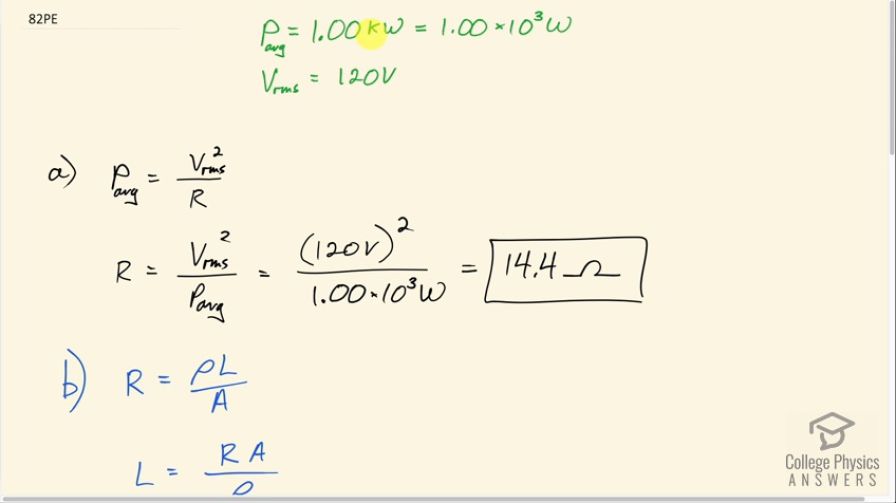
This is College Physics Answers with Shaun Dychko. A radiative heater that has nichrome wires in it to produce heat has an average power output of 1.00 kilowatt, which is 1.00 times 10 to the 3 watts and it runs on a voltage of 120 volts and we assume that that is a rms voltage because this is what's conventional for an outlet in North-America is to state the rms voltage as 120. So that means the average power then is the rms voltage squared divided by the resistance and we are told to figure out what is this resistance? So we multiply both sides by R divided by P avg and then we solve for R is rms voltage squared over average power. So that's 120 volts squared divided by 1.00 times 10 to the 3 watts which is 14.4 ohms. Now in part (b), we are asked what length of nichrome wire would be needed assuming a cross-sectional area of 5.00 square millimeters and an operating temperature of 500 degrees Celsius. So the resistance of a wire is its resistivity multiplied by its length divided by its cross-sectional area. So we know the area and we have to solve for L; we can figure out what the resistivity is as well. So we multiply both sides by area over resistivity and we are left with length then is resistance multiplied by area divided by resistivity so resistance we figured out in part (a) by the way. So the resistivity will be the resistivity we can look up in table [20.1] and that is for nichrome— it is 100 times 10 to the minus 8— but that's the resistivity at 20.0 degrees Celsius. Now we are told that the operating temperature is 500 degrees Celsius and so we need to use this formula which says that resistivity is some known resistivity at whatever temperature, 20.0 degrees in our case, multiplied by 1 plus the temperature coefficient of resistivity multiplied by the change in temperature compared to whatever the temperature was at this known resistivity and the actual operating temperature. So we substitute all of this in for resistivity and we do that in red here and so here's our formula for the length of the nichrome wire: it's gonna be its resistance of 14.4 ohms, as we figured out in the first part, multiplied by 5.0 square millimeters, which we have to convert into square meters by multiplying by 1 meter for every 1000 millimeters and we do that multiplication twice because it's millimeter squared and divide that by the reference resistivity or this value given to us in the table at 20.0 degrees Celsius— 100 times 10 to the minus 8 ohm-meters— multiplied by 1 plus the temperature coefficient of resistivity which we look up in table [20.2] and for nichrome, it's 0.4 times 10 to the minus 3 per Celsius degree so that tells you how quickly the resistivity changes with temperature then we multiply by the change in temperature. So it's 500.0 degrees Celsius—operating temperature, the question tells us— minus the 20.0 degrees that this resistivity was measured at and all of this works out to 60.0 meters is the length of the wire. Part (c) asks what power will this heater draw when it's first switched on and the reason that will be different from its regular power output is because the temperature when it's switched on will be at 20.0 degrees Celsius and its resistivity of the nichrome wire will be different, it will be 100 times 10 to the minus 8 at 20.0 degrees Celsius, assuming that's around room temperature when the heater is switched on. So this initial resistance then is the resistivity at the room temperature, which we'll take to be 20.0 degrees multiplied by the length that we found in part (b) divided by the cross-sectional area. And so we are going to divide the voltage squared by this fraction which is the same as multiplying by its flipped version, its reciprocal, and so we are going to multiply by A over ρ naught L. So the initial power output then is 120 volts squared times the cross-sectional area, written in meters squared, divided by 100 times 10 to the minus 8 ohm-meters— which is the resistivity when it's first switched on— times 60.403 meters—its length, which has extra digits here to avoid intermediate rounding error— and then that works out to 1.2 times 10 to the 3 watts, which is significantly higher than its average power when it's at operating temperature... it's about 20 percent higher. And there we go!