Question
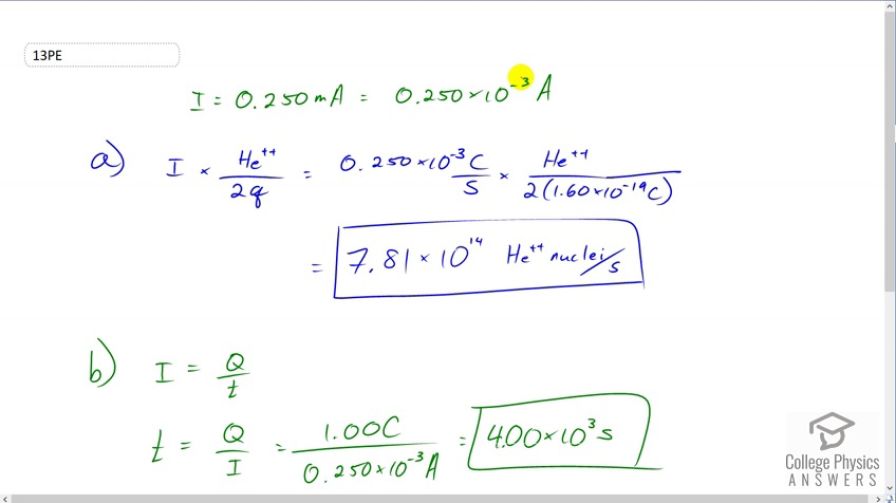
A large cyclotron directs a beam of nuclei onto a target with a beam current of 0.250 mA. (a) How many nuclei per second is this? (b) How long does it take for 1.00 C to strike the target? (c) How long before 1.00 mol of nuclei strike the target?
Final Answer
a)
b)
c)
Solution video
OpenStax College Physics for AP® Courses, Chapter 20, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're given that the cyclotron has a current of 0.25 milliamps which we convert into amps by multiplying by ten to the minus three here which is what the prefix milli means. The next step is to realize that this current has units of coulombs per second and we want to know how many helium nuclei are passing per second. So since we have coulombs per second we've taken care of the units in the denominator already just by writing down the current. Then we'll have to get rid of this coulombs and replace it with number of helium nuclei. So we know there is one helium nucleus for every two times the elementary charge because this is a plus plus here, meaning that there are two electrons stripped away from the helium nucleus leaving two protons behind. So the magnitude of the charge on the helium nucleus is two times the elementary charge. So we take this number and divide it by two times 1.6 times ten to the minus nineteen coulombs, and we end up with 7.81 times ten to the fourteen helium nuclei per second. Then part B, it says how long does it take for one coulomb to strike the target in the cyclotron? Well, we have to rearrange this formula for current and solve for t by multiplying both sides by t over I. We get t is the amount of charge divided by the current. So that's one coulomb divided by 0.25 times ten to the minus three amps which is four times ten to the three seconds. Then the next question in part C is how long would it take for a mole of charge to hit the target? So we have to convert this into coulombs basically, and so we have one mole and we convert that into the number of helium nuclei by multiplying by 6.02 times ten to the twenty-three helium nuclei per mole, this is Avogadro's number, and then times by two times 1.6 times ten to the minus nineteen coulombs for every helium nucleus. So these units cancel and we're left with coulombs on the top. We divide that by the current and we get 7.71 times ten to the eight seconds which is about 24.4 years.

