OpenStax College Physics for AP® Courses, Chapter 20, Problem 12 (Test Prep for AP® Courses)

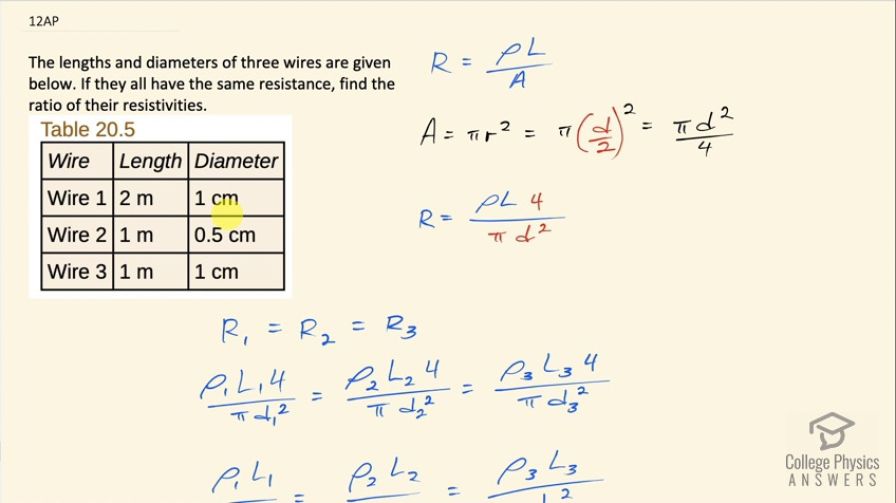
This is College Physics Answers with Shaun Dychko. Three wires all have the same resistance and they have different lengths and diameters and their question is to figure out the ratio of their resistivities. So resistance in terms of resistivity is the resistivity ρ multiplied by the length of the wire divided by its cross-sectional area and we assume that the cross-section is a circle so its area is π times its radius squared but we are given diameter so we have to substitute in diameter divided by 2 in place of radius, this works out to π times diameter squared over 4 is the cross-sectional area of the wire. So we substitute that in place of A but instead of dividing a fraction by a fraction, I prefer to multiply by the reciprocal of the denominator so I am going to flip over this fraction here and multiply ρL by 4 over πd squared. So we can say the resistance of wire one is the same as resistance of wire two and wire three because they are all the same and we can substitute in terms of each wire this formula. So we have resistivity of wire one multiplied by the length of wire one times 4 over π times the diameter of wire one squared equals the same formula with their respective wire numbers as subscripts for the different factors. So for wire two, we have resistivity two, length two and diameter two and wire three has the subscript 3. Now 4 over π is a common factor on all these expressions and so we can multiply everything by π over 4 and then multiply this by π over 4 and multiply this by π over 4 and then we get a cleaner version of this expression so ρ 1L 1 over d 1 squared equals the same expression with their respective wire here. So we want to find ratios of the resistivities so we need to create an expression for two of the resistivities in terms of the other one so the way I have chosen to do it is I am going to write each resistivity in terms of resistivity number one so I am going to have an expression for resistivity two in terms of resistivity one and we will get an answer there and then we'll have resistivity three in terms of resistivity one and then we'll create a ratio after that. So we are going to focus only on this portion here for the moment and solve for ρ 2 by multiplying this by d 2 squared over L 2 and then multiply this by d 2 squared over L 2 and we get this line here. So resistivity two is resistivity one times length one times diameter two squared over diameter one squared times length two. So we have ρ 1 times the length one, which is 2 meters times diameter two, which is 0.5 centimeters and we square that and it's a bit strange to mix meters and centimeters in the same formula but we can get away with it just because we have canceling of the same units on the top and bottom of this fraction so where I have centimeters squared on the top, there's also a corresponding factor centimeters squared on the bottom and so the centimeter squared's will cancel and likewise these meters will cancel and we will be left with a dimensionless fraction and you know, if you are not comfortable with that, you could write 0.5 times 10 to the minus 2 meters— that would be fine as well— because there is a good habit of having meters, kilograms and seconds in our formulas and if you want to continue with that habit, carry on that's a great habit but I just can see that, you know, to save a bit of writing we can get away with using centimeters and meters mixed up together because they are going to cancel. Okay! So we are dividing by diameter of wire one 1 centimeter squared times the length of wire two, which is 1 meter and there we go... this works out to ρ 1 divided by 2 so ρ 2 is ρ 1 over 2 and that's what we wanted and an expression for resistivity two in terms of resistivity one. Then we will look at creating an expression for resistivity 3 in terms of resistivity number 1. So we are going to look at this equals this and solve for ρ 3. So ρ 3 is going to be resistivity one times L 1 times d 3 squared over d 1 squared times L 3 on the bottom here and this is 2 meters times 1 centimeter squared over 1 centimeter squared times 1 meter, this works out to ρ 3 is 2 times ρ 1. And then we are going to set up our ratio of resistivity one to resistivity two to resistivity three and we just have to pick a number for resistivity one and then write what resistivities two and three would be; you could choose any number but tradition is that you should choose that such that at least one of these these resistivities is the number one— that's reducing a fraction in other words. So if we choose the number two for resistivity one, resistivity two is half of resistivity one and 2 divided by 2 is 1 so that's going to make this a reduced ratio and resistivity three is 2 times resistivity one so it works out to 4 so our ratio is 2 to 1 to 4.