OpenStax College Physics for AP® Courses, Chapter 20, Problem 34 (Problems & Exercises)

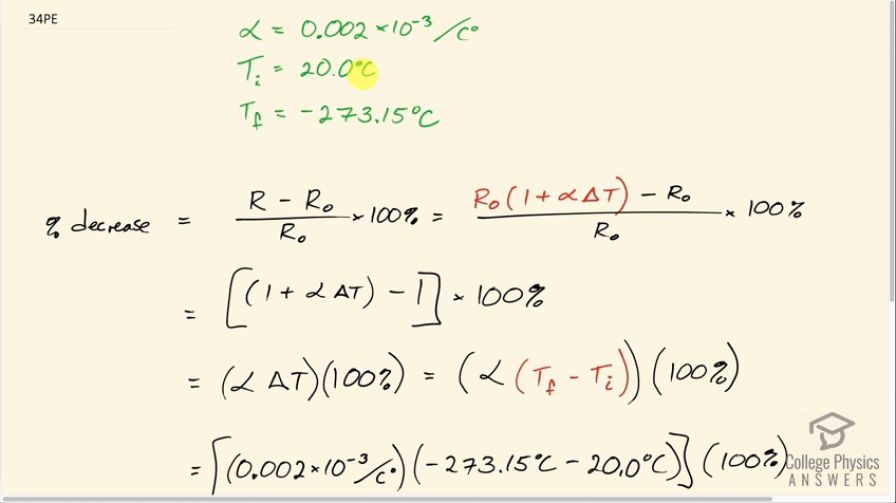
This is College Physics Answers with Shaun Dychko. A wire that's made out of material called constantan starts at a temperature of 20.0 degrees Celsius and we are asked to figure out what is the maximum possible percent decrease in resistance that can be achieved for this wire? So we look up its temperature coefficient of resistivity and see that it is 0.002 times 10 to the minus 3 per Celsius degree and since this is a positive temperature coefficient of resistivity that means that if you look at this formula for how resistance changes with changes in temperature, having α be positive means that as temperature decreases, the resistance will decrease or looking at it from the other perspective, as temperature goes up, multiplying some positive change in temperature by a positive α would result in an increase in resistance. So to decrease resistance of this wire made out of constantan, we need to decrease temperature. The opposite will be true for a wire made out of carbon, germanium or silicon because they have negative temperature coefficients of resistivity. Okay! So to get the maximum possible decrease in resistance, we will have to use the maximum possible decrease in temperature which at its minimum is going to be minus 273.15 degrees Celsius— that is absolute zero— so we will take that to be our final temperature. So the maximum possible percent decrease in resistance then is the difference in the resistance... the new resistance after the temperature has been reduced minus the original resistance R naught divided by R naught times 100 percent. So substituting this formula in place of R to find how the resistance changes with temperature, we have the original temperature R naught at 20.0 degrees Celsius times 1 plus the temperature coefficient of resistivity, α, multiplied by the change in temperature and the R naught cancels everywhere turning into a 1 here because we are dividing each term by R naught. And so we have 1 plus αΔT minus 1 times 100 percent and this 1 minus 1 makes 0 for those terms and we are left with αΔT times 100 percent. ΔT is the final temperature minus the initial temperature and so we have 0.002 times 10 to the minus 3 per Celsius degree—that's α—multiplied by negative 273.15 degree Celsius—the final temperature at absolute zero— minus the initial temperature of 20.0 degrees Celsius times a 100 percent gives negative 0.06 percent is the maximum possible percent decrease in resistance that can be achieved for a wire made out of constantan that starts at 20.0 degrees Celsius.