Question
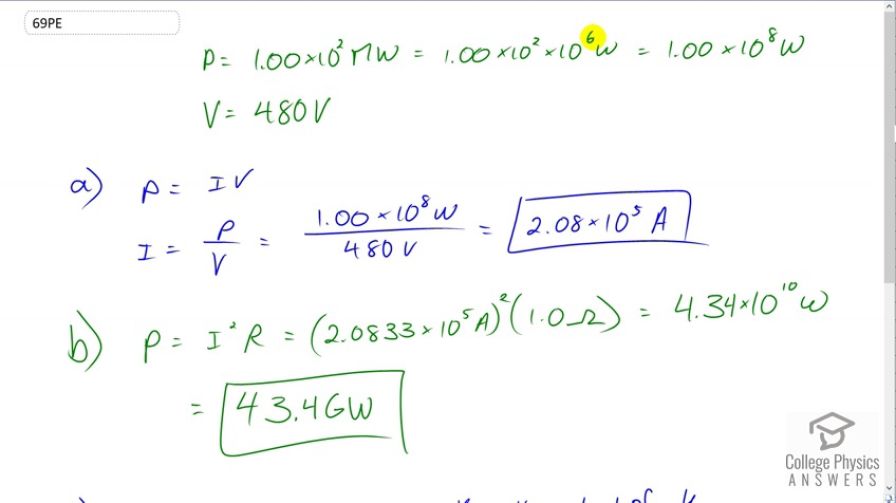
(a) What current is needed to transmit of power at 480 V? (b) What power is dissipated by the transmission lines if they have a resistance? (c) What is unreasonable about this result? (d) Which assumptions are unreasonable, or which premises are inconsistent?
Final Answer
- This power wasted is more than the output of the power plant, and it's wasted by dissipation in the wires.
- The voltage is too low for transmission of this much power.
Solution video
OpenStax College Physics for AP® Courses, Chapter 20, Problem 69 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The alleged power output of this power plant is one times ten to the two Mega Watts, which is one times ten to the two times ten to the six Watts, replacing the prefix Mega with times ten to the six and then in total this makes one times ten to the eight Watts. And the voltage is 480 Volts. So, the-- the current needed to transmit this much power at that voltage, we figure out from P equals IV. And we divide both sides by V to solve for I. So the current is the power divided by the voltage. So it’s one times ten to the eight Watts 480 Volts, which means we would need 2.08 times ten to the five Amps. Now, if wires were carrying that much current and the wire had a resistance of one ohm, the power dissipated within the wire, you know, before it even got to the place-- you know, the city that it’s-- the power plant is delivering power to, it would dissipate 4.34 times ten to the ten Watts, which is 43.4 Giga Watts, which is a huge amount. And that’s more than in entire output of the power plant. And so, this is-- these are not realistic numbers. The voltage is too low for transmission of this much power. So in reality this much power would be transmitted at much much higher voltages in order to decrease the current that is going through the lines in order to reduce the power dissipated within the lines.
