Question
The axis of Earth makes a angle with a direction perpendicular to the plane of Earth’s orbit. As shown in Figure 10.41, this axis precesses, making one complete rotation in 25,780 y.
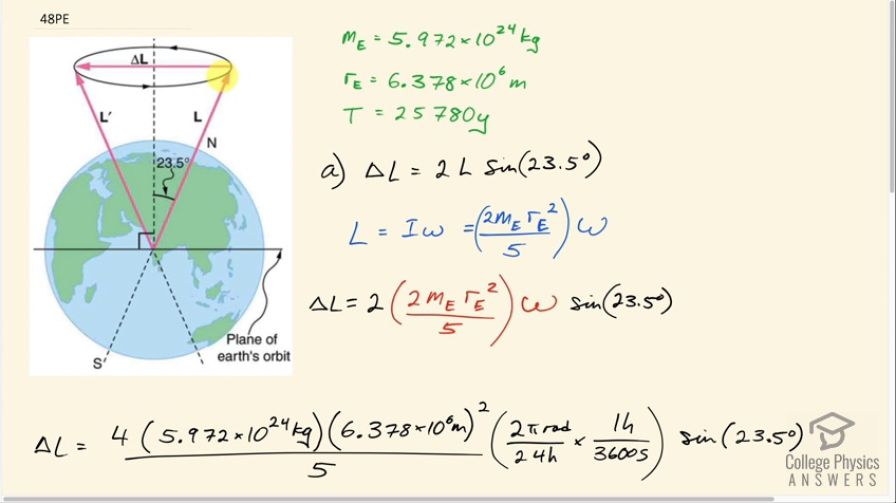
(a) Calculate the change in angular momentum in half this time.
(b) What is the average torque producing this change in angular momentum?
(c) If this torque were created by a single force (it is not) acting at the most effective point on the equator, what would its magnitude be?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 48 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The axis of the Earth's rotation has procession that has a period of 25780 years. So this axis of rotation will be pointing in a slightly different direction going around in a circle once in this many years and the question in part (a) is to find the change in angular momentum. So angular momentum is a vector and if we were to grab the Earth with our right hand, our thumb would be pointing in the direction of this angular momentum and this is one-time and then half of a period later, it gets here so this is, you know, whatever half of 25780 years is it will be pointing over here and this makes a triangle which is symmetrical about this vertical line and so if we find this segment of ΔL, we can just multiply it by 2 and that will give us the full ΔL which is the answer to part (a). So we are gonna find this leg of this right triangle here where L is the hypotenuse and this angle here is 23.5 degrees and there's a right angle up here. So that means ΔL is gonna be 2 times this leg which is L—the hypotenuse— multiplied by sin 23.5 degrees. And L is the moment of inertia of the Earth multiplied by its angular velocity and the Earth is a solid sphere so that means we can use this formula 2mr squared over 5 for its moment of inertia and then multiply that by ω. So ΔL then is 2 times this moment of inertia times ω times sin 23.5 degrees and then the 2 times 2 makes 4 multiplied by the mass of the Earth multiplied by the Earth's radius squared divided by 5 multiplied by one full revolution which is 2π radians every 24 hours times by 1 hour for every 3600 seconds— that gives us radians per second here— and then times sin 23.5 degrees and that's 5.64 times 10 to the 33 kilogram meters squared per second. So that's the answer to part (a). And then in part (b), it's asking what torque would cause this to happen? So torque is the change in angular momentum divided by time and so we have this change in angular momentum divided by time which is one-half of this full period because the angular momentum went from here to here—halfway around— so we are gonna be taking half of T. That's one-half times 25780 years converted into seconds and we have 1.39 times 10 to the 22 newton meters. And what force would cause this torque supposing it was exerted at the equator perpendicular to the radius of the Earth? In that case, well, the torque would be the force times the radius then and we divide both sides by r and we get the force is 1.3855 newton meters divided by 6.378 times 10 to the 6 meters— radius of the Earth— which is 2.17 times 10 to the 15 newtons.

