Question
How do you arrange these objects so that the resulting system has the maximum possible moment of inertia? What is that moment of inertia?
Final Answer
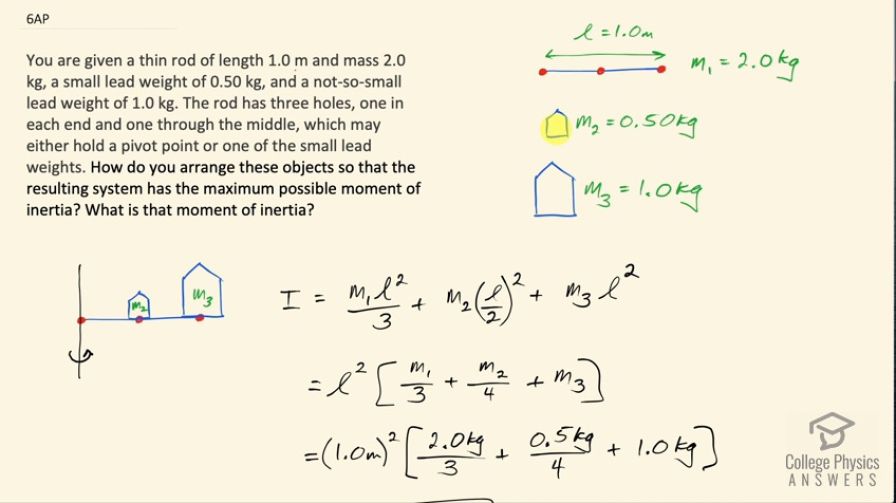
The pivot should be at one end, the most massive mass at the other, and the small mass in the middle.
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 6 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are given a rod of length 1.0 meters and it has a mass of 2.0 kilograms and we are given a point-mass we'll say of mass 0.50 kilograms and another point-mass of 1.0 kilogram so I have labeled these m 1, m 2 and m 3 and the question is how do we arrange these things such that we have the greatest possible moment of inertia and we are told that this rod has three points on it that can be used either for holding a single mass or for holding the pivot. So the arrangement with the greatest moment of inertia is this one here where we have the axis of rotation in an end of the rod so we put the pivot at one end and we put mass two in the middle and mass three at the far end, far from the pivot and the first question to ask is why should the pivot be at an end? Well, we can look at these formulae for moments of inertia for a thin rod and we can either have the axis in the middle in which case the moment of inertia would be the rod mass times its length squared over 12 or we can have the axis at an end in which case, it's mass of the rod times the length squared divided by 3. So these two equations are the same except for the denominator so we'll choose the one that has the smaller denominator in order to have the greatest question. So we are choosing an axis at the end of the rod and it will have a formula mass of the rod l squared over 3. Okay! So that's this term here for the moment of inertia: we are gonna add up the moment of inertia of each of the three things, the three masses here. Mass two is a point-mass and so its moment of inertia formula will be m times r squared, where r is the distance from the axis of rotation and in this case that's the rod length over 2 because it's in the middle of the rod so we have l over 2 all squared times m 2. And then mass 3 is at the end so its moment of inertia will be m 3 times its distance from the pivot, which is the length of the rod squared. We factor out l squared from each term, square this denominator 2 to make a denominator 4 here and we have l squared times m 1 over 3 plus m 2 over 4 plus m 3 and by the way, we chose to have m 2 in the middle and m 3 at the end because we want to multiply this l squared by the largest possible factor and we can either choose to multiply by m 2 or m 3 and it's better to multiply this big number by the other biggest number we can find, which is mass 3 so mass 3 should go in the end and mass 2 should go in the middle. So the moment of inertia then is 1 meter squared times 2 kilograms—mass of the rod—divided by 3 plus 0.5 kilograms divided by 4 plus 1.0 kilogram and that is 1.8 kilograms meters squared.
