Question
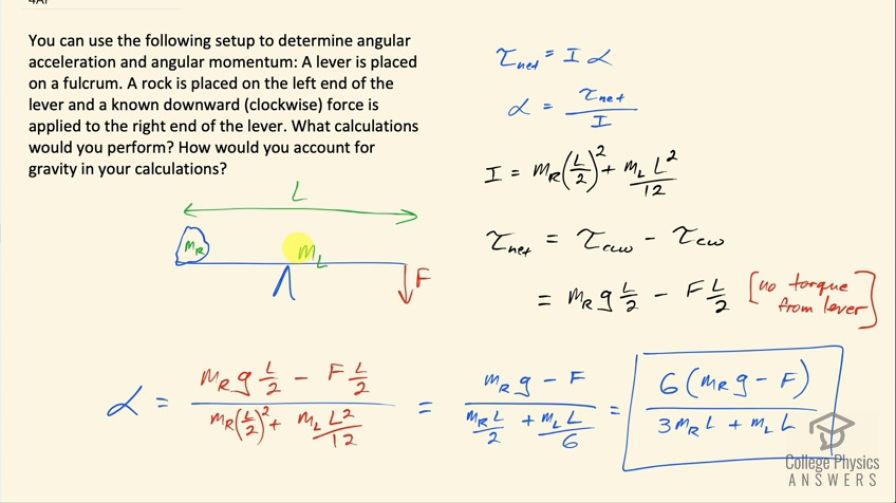
You can use the following setup to determine angular acceleration and angular momentum: A lever is placed on a fulcrum. A rock is placed on the left end of the lever and a known downward (clockwise) force is applied to the right end of the lever. What calculations would you perform? How would you account for gravity in your calculations?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 4 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. A lever is placed on a fulcrum and the fulcrum is positioned in the middle of the lever; the lever has a mass of m L for 'lever' and a rock is placed on one end— the left-end— and I have labeled that mass m R; the lever has some known length L and there's a known force being applied downwards on the right-hand end of the lever and the question is how to find the angular acceleration and angular momentum of this situation? So initially if it's not moving, there's no angular momentum but we will find its angular acceleration. So we know that the net torque on the system equals its moment of inertia times the angular acceleration— this is the angular analog to Newton's second law F net equals mass times acceleration— and we'll divide both sides by I to find α, the angular acceleration. So the angular acceleration is the net torque divided by the moment of inertia. So we need to find the moment of inertia of the system and that consists of two masses: one is a point mass, the formula for which is mass times its distance from the pivot squared and the distance from the pivot is half the length of the lever and so we square that that's the moment of inertia of the rock and then we add to that the moment of inertia of this what we assume is a uniform rod and so formula for that is mass of the rod times its length squared divided by 12— that's the moment of inertia for a rod with an axis in the center of it. So we have mass of the lever times the lever's length squared divided by 12 here. And then we have to find the net torque on the system when there's two sources of torque: one is the force applied at this end and the other is the force of gravity on the rock and the gravity on the lever exerts no torque because the fulcrum is positioned in the middle and so there's no lever arm for the force of gravity on a lever and so there's no torque due to the gravity on the lever. So the net torque is the counterclockwise torque minus the total clockwise torque; the counterclockwise torque is due to the rock and so we have the force of gravity on the rock is mass of the rock times g times its distance from the pivot, which is the lever length over 2 and we subtract from that the clockwise torque, which is the applied force which we assume is perpendicular to this lever times its distance from the pivot, which is also L over 2 and I already mentioned there's no torque due to mass of the lever. Okay! So then we substitute into this formula for angular acceleration and we have the net torque, which we have copied here divided by the angular... or sorry, the moment of inertia I should say which I have copied from there to here and then multiply top and bottom by 2 over L to simplify it a little bit and that gives this line here m Rg minus F over m RL over 2 plus m L times L over 6 then multiply top and bottom by 6 to clean it up just one last step and we have 6 times mass of the rock times gravitational field strength minus the force all divided by 3 times mass of the rock times the lever length plus mass of the lever times lever length.