Question
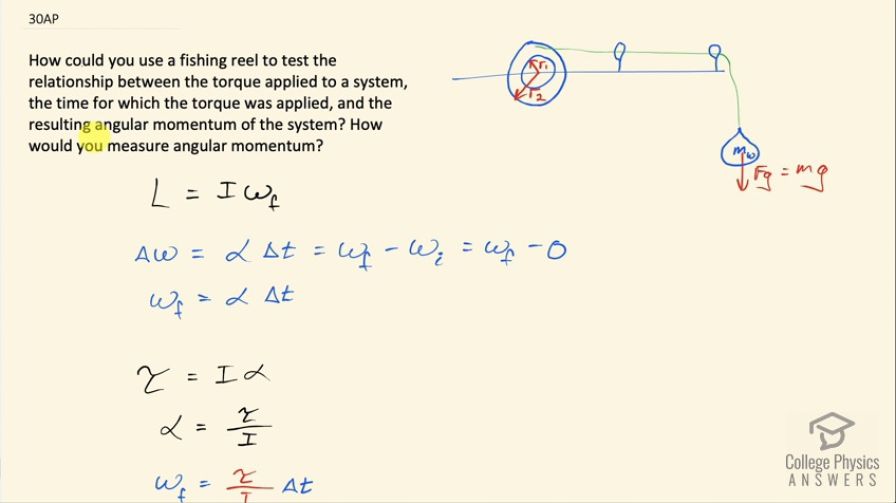
How could you use a fishing reel to test the relationship between the torque applied to a system, the time for which the torque was applied, and the resulting angular momentum of the system? How would you measure angular momentum?
Final Answer
Please see the solution video.
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 30 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We want to use a fishing reel to figure out the relationship between torque applied to the reel and the resulting angular momentum that it will have and we have to figure out how to measure this angular momentum. So angular momentum is moment of inertia times final angular velocity and the change in angular velocity we know is angular acceleration multiplied by time... this also is the final minus initial angular velocities, the initial being zero though so we can say that the final angular velocity then is angular acceleration multiplied by time that gives us something to substitute here but before we do that, we can express angular acceleration in terms of torque because we want to know about torque here. So torque is moment of inertia times angular acceleration and we can divide both sides by I to solve for α and that is the final angular speed then is substituting τ over I in place of α here times Δt and then we can put all of this into our formula for angular momentum and say that it's moment of inertia times τ divided by moment of inertia times time and that means angular momentum is torque times time. The torque here is this known weight... we have to measure the mass of this known weight here hanging from the fishing line and that's going to have some force of gravity on it due to its mass times g and that's the force that's being applied on the reel here perpendicular to the radius the radius that's important here is r 2 and so the torque then is this force of gravity multiplied by r 2 and that's what we say here and so that can be substituted in for torque. And then we can also say that the angular momentum is the moment of inertia of this annular ring and we get that from this formula here we have a ring which has some thickness and so it has some inner radius r 1 and outer radius r 2 so it's m over 2 times r 1 squared plus r 2 squared times the final angular speed and so we can write this in place of L and we do that here and it equals the right-hand side copied there and this force of gravity is the mass of the hanging weight times g and now we can vary the mass that's hanging and see how... because that tells us what the torque is going to be because that's resulting in the weight, which multiplied by the outer radius of the reel and see how the final angular momentum changes with changes in this weight and use our final expression substituting for the final angular speed in place of ω f we can measure the amount of fishing line that is leaving... that's, you know... as its weight drops, we can measure how much fishing line is coming out of this fishing rod and divide that by the circumference of the reel to get the number of revolutions of the reel multiplied by 2π radians for every revolution and then divide by time and that's the final angular speed and here's probably the final expression that we could use for showing a relationship between angular momentum on the left and torque and time on the right and that's what we were expected to talk about is angular momentum on the left and torque and time on the other side.