Question
Which will cause a greater increase in the angular velocity of a disk: doubling the torque applied or halving the radius at which the torque is applied? Explain.
Final Answer
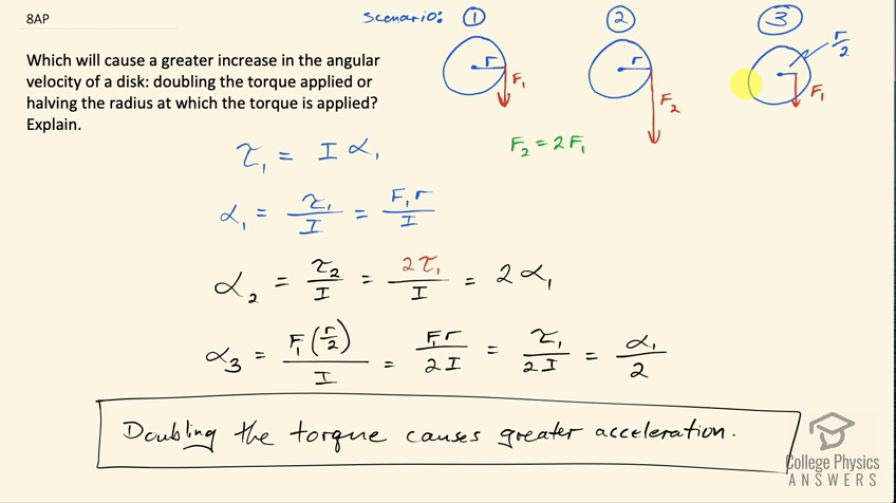
Doubling the torque causes greater acceleration.
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 8 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. I have to say that the wording in this question is a little bit ambiguous I have made an assumption about what they mean and I will explain what that is but it's possible to read other scenarios into this wording as well. Let's take scenario number 1 the original scenario where you have some force we'll call it F 1—applied on the edge of a disk— and some distance r from the center and that will be some torque τ 1 equals the moment of inertia times some angular acceleration 1. We want to find how the angular acceleration changes with these different scenarios because you know, increasing angular velocity is the same as saying having a high angular acceleration. So scenario two is where you double the torque and so let's suppose we have a force acting at the same position as in scenario 1 but the force is twice as large and so that would be an example of doubling the torque and in that case, we have some torque number 2 divided by the same moment of inertia because this is the same disk with the same radius and this torque two we are told is twice what the original torque was and τ 1 over I is α 1 and so we are left with 2 times α 1 so having twice the torque gives twice the angular acceleration. Scenario three says halve the radius at which the torque is applied. So I guess we'll have the same force say but it's going to be applied at a distance half of what it was before from the center and so we have the same force as before F 1 but now its lever-arm is half the radius and this works out to F 1r over 2I and F 1r is the original torque τ 1 and τ 1 over I is α 1 and we end up with half the angular acceleration in scenario three. So doubling the torque causes the greater acceleration.