Question
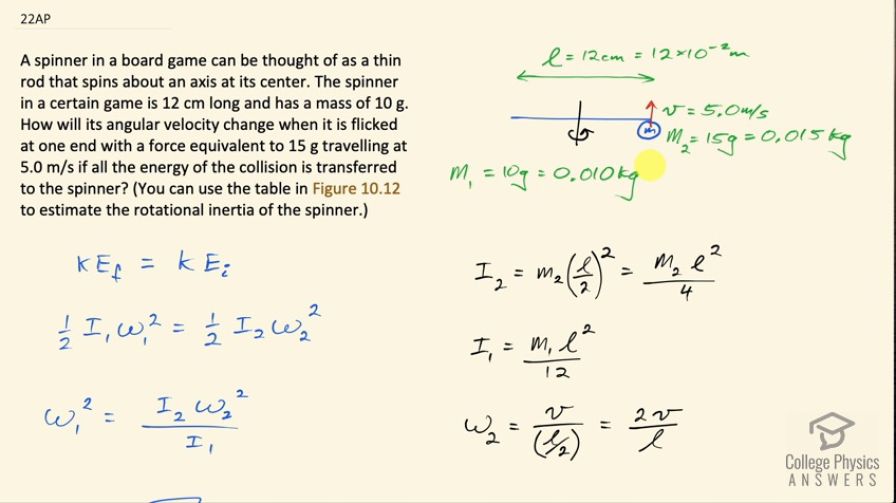
A spinner in a board game can be thought of as a thin rod that spins about an axis at its center. The spinner in a certain game is 12 cm long and has a mass of 10 g. How will its angular velocity change when it is flicked at one end with a force equivalent to 15 g travelling at 5.0 m/s if all the energy of the collision is transferred to the spinner? (You can use the table in Figure 10.12 to estimate the rotational inertia of the spinner.)
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 22 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a board game spinner that has an axis of rotation in the middle and it has a length of 12 centimeters and it's going to be flicked—it's initially at rest we assume— it's going to be flicked with a force equivalent to 15 grams moving at 5 meters per second hitting its end and we assume that of course the velocity is perpendicular to the spinner. So in this collision, all the energy is transferred to the spinner we are told. This problem is a bit different than most other problems that we solve in this chapter because this mass is a hypothetical mass, it's not real it's... I guess, a person's fingertip I suppose that will be taken out of the system immediately after the collision. So what we are doing is we are imagining that the rotational kinetic energy that this hypothetical mass has just at the moment of collision is going to be transferred entirely into rotational kinetic energy of the spinner alone after the collision. And so that's what this is saying is the final kinetic energy equals the initial kinetic energy; the final kinetic energy will just be the rotational kinetic energy of the spinner because like I said this hypothetical mass, you know, is taken out of the system and so our final kinetic energy is one-half times the moment of inertia of the spinner times its angular velocity squared and everything to do with the spinner, I have labeled with the subscript 1 and that's going to equal the rotational kinetic energy that we have initially with this hypothetical mass that's one-half times its moment of inertia— I 2—times its angular velocity— ω 2—squared. Okay! So then we figure out what a lot of these different factors are here. The moment of inertia of the hypothetical mass is that of a point mass so its mass times its distance from the axis of rotation, which is the length of the spinner divided by 2 and that's gonna be m 2l squared over 4. The moment of inertia of the spinner we look up in our table of formulae and that's going to be the mass of the spinner times its length squared over 12— that's for a thin rod with an axis through its center— and then the angular velocity of this mass is going to be its linear velocity divided by its distance from the axis of rotation and this is again kind of a hypothetical angular velocity... I mean this thing we know is not going to be going around in a circle but you know we can imagine that at this particular instant in time, it's moving as though it's going in a circle because it has a velocity perpendicular to the radius just as an object does when it is moving in a circle. So at this particular instant in time of the collision, it's angular velocity is 2 times its linear velocity divided by l. Okay! So multiplying top and bottom here by 2 by the way to get that. There! Well, we multiply both sides of this by 2 divide both sides by I 1 and we end up with angular velocity of the spinner after the collision squared equals moment of inertia of the hypothetical mass times its angular velocity before the collision squared divided by the moment of inertia of the spinner. Then take the square root of both sides and we have ω 1 equals square root I 2 over I 1 times ω 2 to the power of 1. So then we plug in for I 2, it's m 2l squared over 4 and then we are going to divide by this moment of inertia but instead of dividing this fraction by a fraction, I am going to instead multiply by the reciprocal of the denominator so I am multiplying by this flipped over so multiplying by 12 over m 1l squared and then substituting for ω 2 with 2v over l and this simplifies to square root 3m 2 over m 1 and then multiply that by 2v over l. So that's square root of 3 times 0.015 kilograms— mass of the hypothetical mass— divided by 0.010 kilograms of the spinner times 2 times 5.0 meters per second divided by 12 times 10 to the minus 2 meters— length of the spinner— and that's 180 radians per second is the angular speed we expect the spinner to have after the collision... or after the flick.
