Question
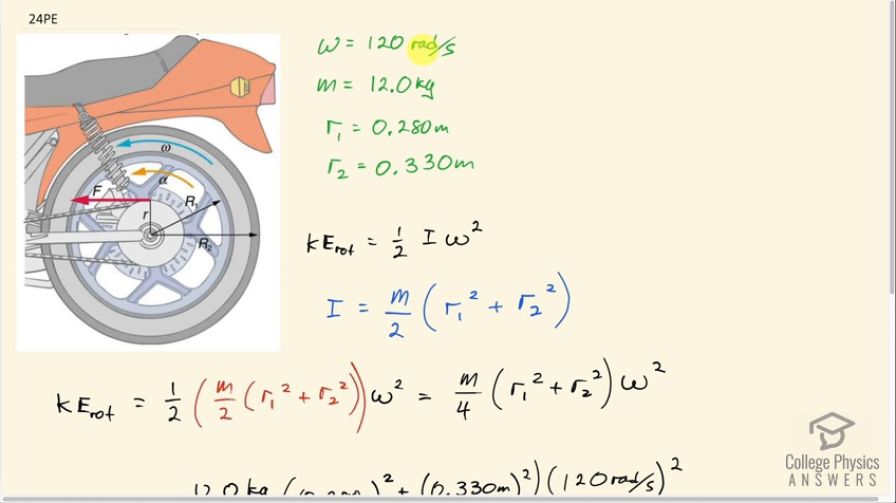
Calculate the rotational kinetic energy in the motorcycle wheel (Figure 10.38) if its angular velocity is 120 rad/s. Assume M = 12.0 kg, R1 = 0.280 m, and R2 = 0.330 m.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 24 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to calculate the rotational kinetic energy of this motorcycle wheel given its angular speed of 120 radians per second, a mass of 12.0 kilograms and then inner radius of 0.280 meters and then outer radius of 0.330 meters. So the rotational kinetic energy is one-half times moment of inertia times angular speed squared and the moment of inertia we look up in our table of formulae here and we have to use this one because this is for when you have a case where this thickness of the cylinder cannot be ignored. So this tire has mass to it that can't be ignored and it's spread out over some radius here. So it's mass over 2 times the inner radius squared plus the outer radius squared so that's what's written here. So we make that substitution for I in this formula for rotational kinetic energy and we have these 2's in the denominator multiplying together to make 4 so we have m over 4 times r 1 squared plus r 2 squared times ω squared. So that's 12.0 kilograms over 4 times 0.280 meters squared plus 0.330 meters squared times 120 radians per second squared giving a kinetic energy of 8090 joules.
