OpenStax College Physics for AP® Courses, Chapter 10, Problem 22 (Problems & Exercises)

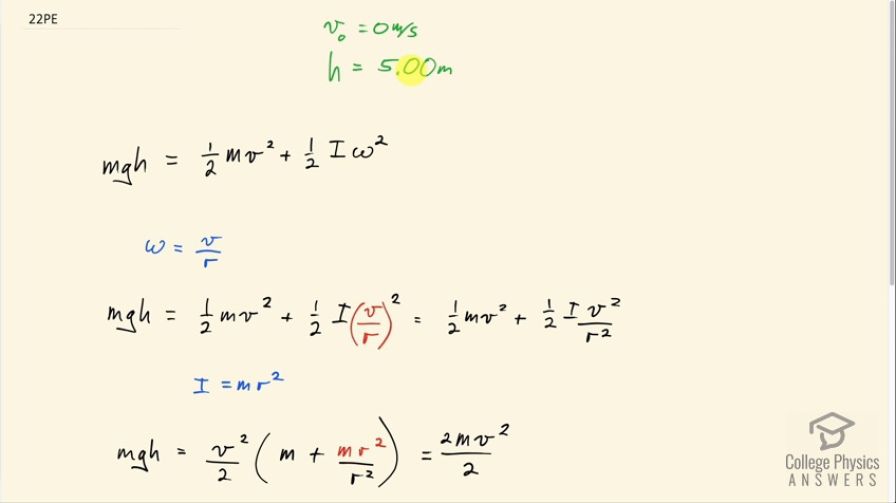
This is College Physics Answers with Shaun Dychko. A hoop is going to start from rest and roll down a hill that is 5 meters high and the question is what will the velocity of the hoop be at the bottom of the hill? Well, the gravitational potential energy that the hoop has initially is gonna get turned entirely into translational kinetic energy and rotational kinetic energy. So this is one-half m translational velocity squared plus one-half moment of inertia times angular velocity squared. So this angular velocity we can replace it with v over r because if we have a hoop we are curious to know what the velocity of this center will be—this is how fast the hoop is moving forwards— and it is going to be the same as the tangental velocity of a point on the edge and that velocity is... well, this angular velocity is given by v over r where you can say the velocity of this point on the edge is r times ω. And then we want to solve for ω and write it as v over r and replace ω with that. So now we have an expression containing only translational velocity so this v got squared and so did the r on the bottom. Then we can make a substitution for the moment of inertia; it's going to be mass times radius squared because if we look at our formulas here, the formula for a hoop is mr squared and we replace I with that and I also factored out the v squared and the denominator 2 from both factors here. So we have m plus mr squared substituting for I divided by r squared—that's just copied from there— and this is 1 and so we have 2m inside this bracket so we have 2m times v squared over 2 equals mgh. So the 2's cancel and we can multiply both sides by 1 over m and then switch the sides around and take the square root of both sides and we get v is square root gh. So that's 9.80 meters per second squared times 5.00 meters square rooted which is 7.00 meters per second.