Question
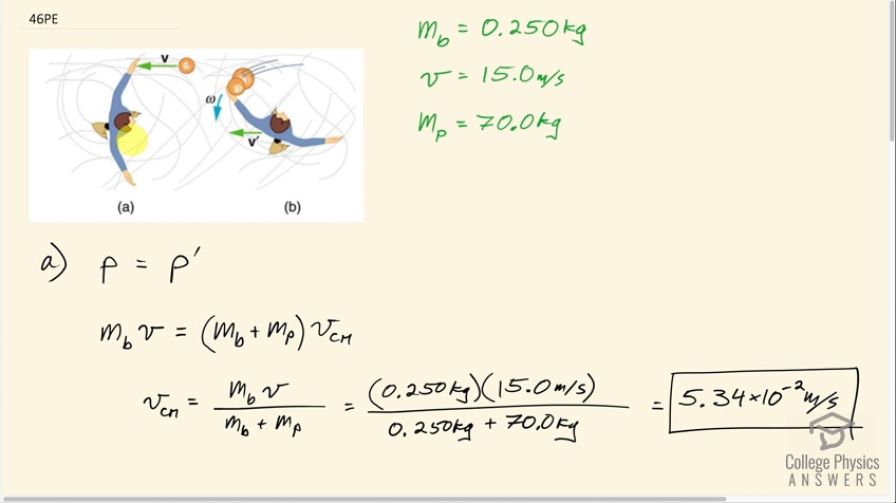
Suppose a 0.250-kg ball is thrown at 15.0 m/s to a motionless person standing on ice who catches it with an outstretched arm as shown in Figure 10.40.
(a) Calculate the final linear velocity of the person, given his mass is 70.0 kg.
(b) What is his angular velocity if each arm is 5.00 kg? You may treat the ball as a point mass and treat the person's arms as uniform rods (each has a length of 0.900 m) and the rest of his body as a uniform cylinder of radius 0.180 m. Neglect the effect of the ball on his center of mass so that his center of mass remains in his geometrical center.
(c) Compare the initial and final total kinetic energies.
Final Answer
- ,
since this collision is inelastic since the ball sticks to the person.
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 46 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This person is initially at rest standing on ice, which is to say that they are standing on a frictionless surface we assume and a ball is incident at a speed of 15.0 meters per second and they are going to catch it and after they catch it, they will end up spinning and also end up translating and we have to figure out what is this v prime—this translation of velocity of this ball-person system after they catch the ball. The mass of the person is 70.0 kilograms and the mass of the ball is 0.250 kilograms and here we go! So the initial momentum of the system is just that of the ball... mass of the ball times its velocity equals the linear momentum of the system after catching the ball and this linear momentum is going to be the velocity of the center of mass which is the velocity of the person, in other words, multiplied by the total mass of the system— ball plus person. So we can solve for this v cm by dividing both sides by the total mass and then switch the sides around and we get the center of mass velocity is mass of the ball times the initial incident velocity divided by the total mass of the ball plus person. So it's 0.250 kilograms times 15.0 meters per second divided by 0.250 plus 70.0 kilograms which is 5.34 times 10 to the minus 2 meters per second and we expect a small answer here... a small number because this person is much more massive than the ball is and so in order for momentum to be conserved, they will have to be moving at a much slower speed to have the same momentum. Part (b) asks what is the angular velocity if each arm is 5.00 kilograms and the arm length is 0.900 meters—measured from the center of their body— and then the radius of their core— I didn't want to use 'b' for body because b has already been used as a subscript for 'ball' so their body—we'll call it the core—has a radius of 0.180 meters. We are going to figure out what is the angular velocity of this person after they catch the ball? So we know that angular momentum before they catch the ball is going to equal angular momentum after they catch the ball. So before they catch the ball, their angular momentum is their moment of inertia multiplied by their angular velocity. Now you have to use your imagination a little bit here... this is just right when they catch the ball and at that particular moment, just at catching the ball, the moment of inertia is just that of the ball because it's not quite in their hand yet so we don't include the body in any of that so it's just a point mass... a distance, you know, length of the arm away from the axis of rotation where the center of mass is... this should be m b by the way— the mass of the ball. Okay! So that's the moment of inertia of just this single ball coming in and its angular velocity is its linear velocity divided by the distance to the axis of rotation although there isn't really any rotation happening quite yet because it's not quite touching the hand but it's just at that cusp between in the hand and not. So here we have... and this by the way is v prime... that's another funny thing... so this velocity that we are using here is not 15.0 meters per second it's not this incident velocity here necessarily, it is the velocity at the center of mass and so we can imagine that you know... when I said this, well, you know, it's only the ball that we have to be concerned with because it's not really in their arm yet but on the other hand, we are talking about v prime and so the center of mass is already moving which of course doesn't happen until the ball is in their hand but this is all just sort of like at that border time and this v prime is going to be the incident velocity of the ball minus the velocity of the center of mass and so this is just immediately before rotating I mean their center of mass could move and the ball could be in their hand before they start actually rotating I think that's the right way to look at it so yeah... this is just moment of inertia of the ball because they haven't started rotating really yet and so here we are but the ball has been caught and so the velocity we are concerned with is the incident velocity of the ball minus the velocity of the center of mass—I mean the center of mass is moving since the ball has been caught. Okay! So we do what we can here. This is after the ball has been fully caught and they are starting to rotate and their angular momentum will be 2 times that of the arm—because two arms— plus the angular momentum of their core plus the angular momentum of the ball and so each of those are going to get multiplied by the same angular velocity—ω prime—that we have to find and we have 2 times the moment of inertia of the arm plus moment of inertia of the core plus moment of inertia of the ball. So each arm is considered a rod with an axis of rotation at the end and so the formula for that is here: mass times length squared over 3 and so that explains where this comes from mass of the arm times length of the arm squared over 3 and there's two of them plus the core which is a cylinder with an axis at the center and so that's this formula—MR squared—over 2 and plus the point mass of the ball multiplied by the distance to the axis of rotation, which is the length of the arm squared. Okay and all that gets multiplied by this ω prime. So all of this here is L prime and that equals the initial L, and so we do that here where I have made a substitution of v minus v cm in place of v prime. Okay! And so then the angular velocity after the ball is caught... I'll make that blue because there's no substitution happening there... is going to be this after we divide both sides by this bracket and then we plug in numbers. So we have 0.250 kilograms times 0.900 meters times 15.0 meters per second minus the answer to part (a) all divided by the total moment of inertia and this works out to 0.868 radians per second. Part (c) asks us to compare the kinetic energy before and after the ball is caught. So before it's caught, it's very straightforward: it's one-half mass of the ball times its velocity squared. So that's one-half times 0.250 kilograms times 15.0 meters per second squared, which is 28.1 joules. The final kinetic energy is going to be the translational kinetic energy due to the center of mass moving plus the rotational kinetic energy. So this is the total mass of the ball plus person multiplied by the center of mass velocity squared plus one-half times the moment of inertia of the whole system times its angular velocity squared. So that's one-half times 0.250 kilograms— mass of the ball— plus 70.0 kilograms—mass of the person— times—their center of mass speed— 5.3381 times 10 to the minus 2 meters per second squared plus the term from their rotational kinetic energy so that's one-half times the total moment of inertia times the angular speed of 0.86798 radians per second squared and this makes 1.56 joules of total kinetic energy after catching the ball. And this is significantly less than the initial total kinetic energy and we expect that since the collision is inelastic because they have caught the ball and some kinetic energy is lost in inelastic collisions.


