OpenStax College Physics for AP® Courses, Chapter 30, Problem 21 (Problems & Exercises)

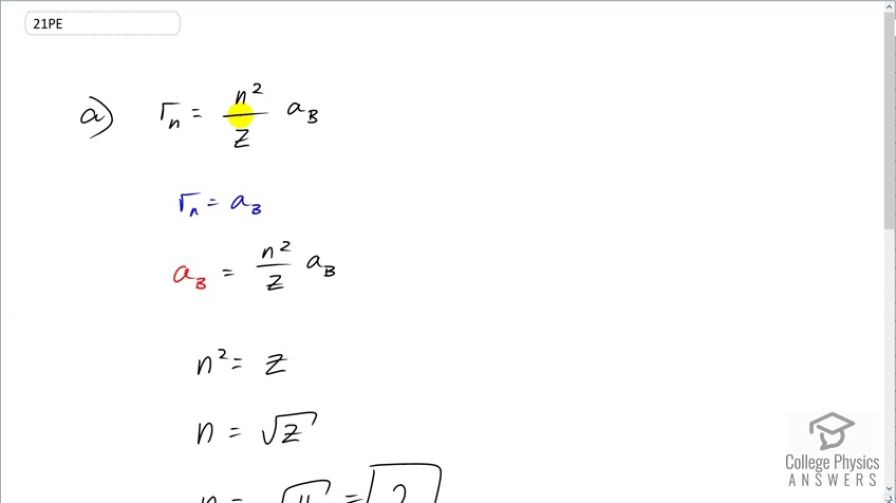
This is College Physics Answers with Shaun Dychko. The radius of a hydrogen-like atom which is to say either a hydrogen atom or an ion with only one electron is the energy level of the electron squared divided by the atomic number or the number of protons, in other words, multiplied by Bohr's radius. Now in this question that we are told that the radius is Bohr's radius; it's the same as the radius of hydrogen atom. And so we can substitute a B in place of r n and then these a B's cancel and so multiply both sides by the atomic number Z and we get n squared equals Z in which case, n, the energy level is square root of Z. And so that's a square root of 4 because beryllium has an atomic number of 4 and that means n is 2. Now we want to know what the energy would be to ionize this ion based on the electron beginning in an energy level of 2. So the amount of energy needed to to ionize it is the difference in the final energy minus the energy that it starts with. Well, the final energy is going to be zero and the initial energy will be some negative number and we have this formula [30.26] which tells us what the energy is of a particular orbit. And so that's gonna be the principal or the atomic number squared divided by the principal quantum number squared or energy level squared, in other words, times ground state energy E naught. Well, E naught is 13.6 electron volts so we have the energy of some state n is negative 13.6 electron volts times the number of protons squared divided by the energy level squared. So the ionization energy then is going to be the final energy of zero once the electron is free of the atom minus negative 13.6 electron volts times the number of protons in the beryllium ion of 4 squared divided by the energy level that it started in which is 2, as we discovered in part (a), squared and this gives 54.4 electron volts of energy to ionize the ion.