OpenStax College Physics for AP® Courses, Chapter 30, Problem 50 (Problems & Exercises)

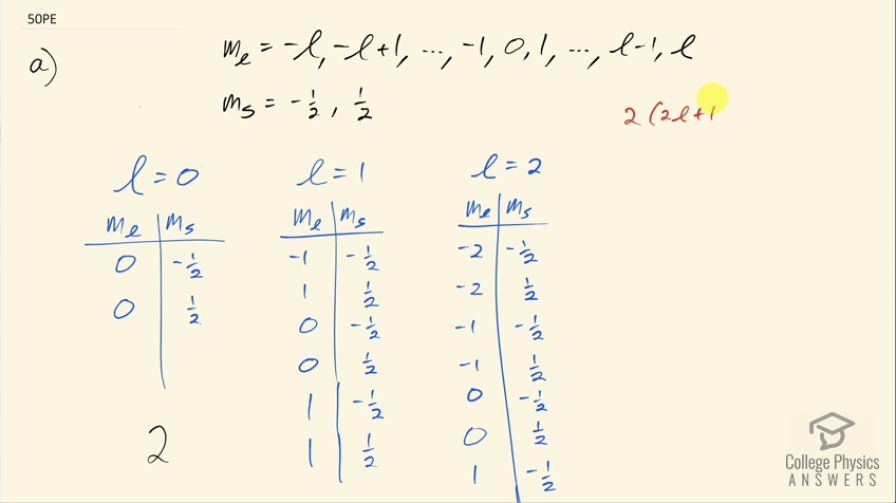
This is College Physics Answers with Shaun Dychko. We are going to prove that the maximum number of electrons in a sub-shell is 2 times 2l plus 1, where l is the angular momentum quantum number, it is the number that designates a sub-shell so for a single value of l, the maximum number of electrons in that sub-shell designated by the value for l is 2 times 2l plus 1. Okay! So let's look at how the other quantum numbers are constrained given a certain value for l; this is the angular momentum projection quantum number and it goes from negative l up to l in integers and then there is the spin projection quantum number, which is always negative of a half or positive of a half. So given l equal to 0, let's count up the number of electrons and then we'll try l equals 1 and l equals 2 and then we'll try to identify a pattern in the sequence that we get. So for l equal to 0, m l can only be 0 in which case you can have two possible states or 2 electrons since for this value of l, you have m l equal to 0, you will have two values that are possible for the spin projection quantum number. For l equal to 1, you can have the angular momentum projection quantum number— negative 1, 0 or 1— and for each value of m l, you can have two values for m s and this is a total of six possible states and then for l equal to 2, m l can go from negative 2 up to positive 2 and for each value of m l, you have two values of m s and this gives a total of ten rows here— that is ten possible states— and so our sequence is for l equal to 0, 1 or 2, we can have a number of electrons equal to 2, 6 or 10 and so on but I think we can establish a pattern just with these three terms. This is an arithmetic sequence because each term you can calculate by adding 4 to the previous term so there's a common difference between terms 6 minus 2 is 4 and 10 minus 6 is 4; you get the same difference between each consecutive term so that is what makes it an arithmetic sequence and that means we take the first term plus the common difference multiplied by the term number so this is the first term, the second term and the third term. Strictly speaking, it's the term number minus 1 but l already is the term number minus 1 so this is the first term but we want to value 0 there and l is 0 so that's nice and so we have 2 plus the common difference times l and if you factor out a 2, you get this formula here which is 2 times 2l plus 1. Okay! Just to expand on this idea of the general term from the arithmetic sequence is the first term plus the common difference— I am not sure what letters you guys are using in your math classes so I am just writing words— common difference times the nth term minus 1 or the term number minus 1, where this is the first term, the second term and the third term. Okay! So then in part (b), we have to figure out the maximum number of electrons in a shell so a given value of n in other words. So for a given value of n, you can have a bunch of different values for l and for each value of l, you will get number of possible number of electrons. So the number of electrons in a particular shell, which is a certain value of n, is going to be 2 for l equal to 0 plus 6 for l equal to 1 plus 10 for l equal to 2 plus so on and so on until you get to this maximum value and this is this formula where l is replaced with n minus 1 because that's the maximum value that's possible for the angular momentum quantum number the maximum it can be is the shell number minus 1. This is an arithmetic series and the word 'series' here means you have to add them all up and these plus signs are what distinguish this from what you see up here this is a sequence because there's commas listing separating each of these terms whereas here we are meant to add up all of these terms into a total. So there's a formula that conveniently helps us find the sum of an arithmetic series and that is you take the first term and add it to the last term and then multiply by the number of terms divided by 2. So the first term is 2, the last term is 2 times 2 times n minus 1 plus 1 so that's what's been substituted here and then the number of terms is going to be n and then we divide that by 2 because the formula says so here— the derivation of this formula is quite neat but that's probably a subject for another video. So the number of terms is n because we are counting these terms starting from zero so the value of the last term is going to be 1 less than the number of terms so if n was 5 for example, in which case the last value is 4 there would be five terms because there would be 0 as a first, 1 as the second and then and then 2 would be the third, 3 would be the fourth and this number 4 would be the fifth term corresponding to n equal to 5 so n is telling us the number of terms. And so this works out to well, one step leads to another I guess you multiply this 2 into the bracket here and you have 2n minus 2 and then the negative 2 plus 1 makes negative 1 here and then multiply through by 2 here, you have 4n minus 2 and then this is 2 minus 2 is zero and you have 4n times n over 2, which is 2n squared. So the maximum number of electrons in a shell, which is a specific value of n, is 2n squared.