OpenStax College Physics for AP® Courses, Chapter 30, Problem 59 (Problems & Exercises)

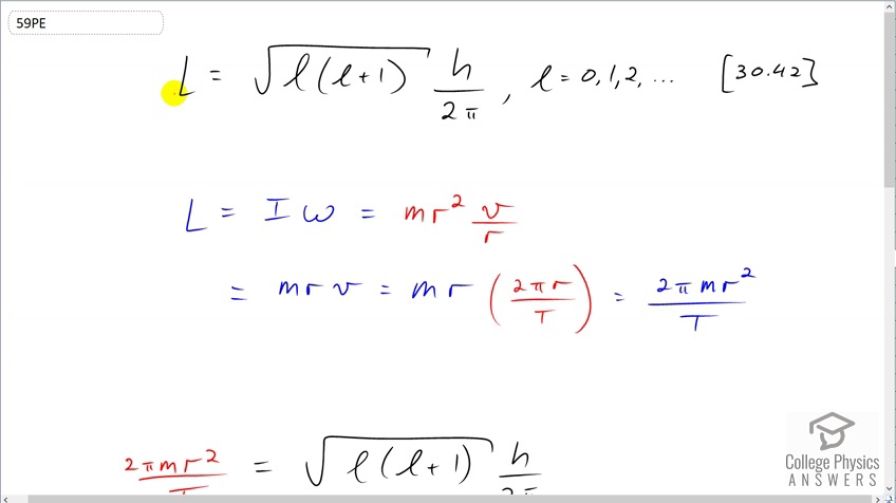
This is College Physics Answers with Shaun Dychko. We are going to figure out what the angular momentum quantum number is for the moon orbiting around the Earth. So the angular momentum is the square root of the momentum quantum number times that plus 1 times Planck's constant over 2π and the angular momentum can also be thought of in a macroscopic context as the moment of inertia times the angular velocity. So the moon is a point mass going around the Earth and so it's moment of inertia is mr squared— that's mass times its orbital radius squared— and the angular velocity of the moon is its tangental velocity divided by its radius of its orbit and r squared divided by r makes r. So the angular momentum then is mrv and v is the circumference of its orbit divided by its periods so this is the distance that it travels divided by the time it takes to do one circle. So this is the size of the circle divided by the time it takes to do one circle and that's its tangental velocity. And so we know what the orbital period is of the moon. And so this makes 2π times m r squared over the period T—that's the angular momentum. So we substitute this in for l here and that's shown here. And now we'll get the l isolated on one side by multiplying both sides by 2π over h and then we'll also make an assumption or an approximation that square root of l times l plus 1 is the same as square root of l squared when l is really large. So if l is really large, adding 1 to it will make no significant difference if the number is already super large I think 1 doesn't change it in any appreciable way. So this is the same as l essentially and then multiply by this l makes l squared the square root of which is l. So we can replace all of this with l here and then at this point, I go h over or 2π times or divided by h on both sides. OK. So l is 4π squared m r squared over h times T and then we plug in numbers. So that's 4π squared times the mass of the moon— 7.348 times 10 to the 22 kilograms— times the orbital radius on average—3.844 times 10 to the 8 meters—squared divided by Planck's constant times the orbital period of the moon which is 27.32 days but we have to write this in units of seconds in order to have mks units—meters, kilograms and seconds— so we multiply that by 24 hours per day times 3600 seconds per hour and we end up with 2.74 times 10 to the 68 is the angular momentum quantum number equivalent for this Earth-moon system.