OpenStax College Physics for AP® Courses, Chapter 30, Problem 62 (Problems & Exercises)

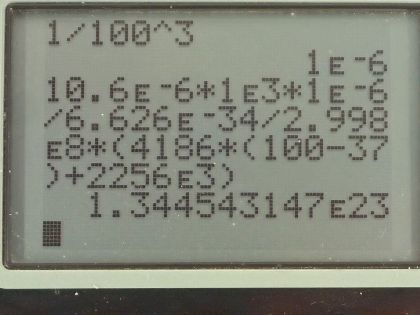
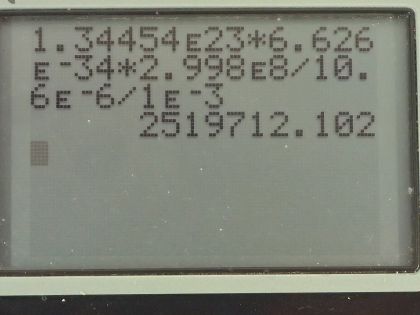
This is College Physics Answers with Shaun Dychko. A carbon dioxide laser that has a wavelength of 10.6 micrometers is being used to ablate some flesh, some skin tissue and ablate means to zap it and increase its temperature to the point where it vaporizes and it's going to do so in 1.00 millisecond and it's going to vaporize 1.00 cubic centimeter of flesh which when we convert into cubic meters by multiplying by 1 meter for every 100 centimeters three times that's 1.00 times 10 to the minus 6 cubic meters. We are going to assume that the skin begins at a temperature of 37.0 degrees Celsius—that's body temperature— and it will have a final temperature of 100 degrees Celsius before it boils away and it has a specific heat of 4186 joules per kilogram per Celsius degree since we are told to assume that the flesh has the same properties as water so this is the specific heat of water and here's the latent heat of vaporization 2256 times 10 to the 3 joules per kilogram and I found both these pieces of information in tables [14.1] and [14.2]. So here's the specific heat of water and then here's water's latent heat of vaporization. Okay! So part (a) is asking how many photons are needed to do this? So the total energy from some number of photons will be the number of photons multiplied by the energy per photon and the energy per photon is Planck's constant times speed of light divided by the photon wavelength. This energy is going to be providing the energy to raise the flesh's temperature and then also to vaporize the flesh. So this is mass times specific heat times change in temperature is the amount of energy needed to raise the temperature and then we have this term—mass times latent heat of vaporization— is the amount of energy needed to change the state from liquid to vapor when it has finally reached 100 degrees. We can factor out m and we have m times cΔT plus L v. So this is the energy needed to be supplied by the light and that's going to be equal to this here so we have Nhc over λ is equal to this and then we have to solve for N— the number of photons. We'll multiply both sides by λ over hc so we have the number of photons then is the wavelength times the mass divided by Planck's constant times speed of light times the specific heat and this c is different from this c so sorry if that's confusing but these are the letters that are used and this is kind of a collision of two formulas from two different domains of physics that happen to have chosen the same letter for different things. So we are multiplying the specific heat by change in temperature plus latent heat of vaporization. Now we don't know what the mass is but we know that the density of the skin will be approximately the same as of water suppose and that density will be the mass divided by the volume and we do know the volume so we'll solve for mass by multiplying both sides by v. So the mass is density times volume so we substitute that in for m here and then we plug in a whole bunch of numbers. So we have 10.6 times 10 to the minus 6 meters is the wavelength multiplied by the density of the flesh— 1.00 times 10 to the 3 kilograms per cubic meter— times the volume of 1.00 times 10 to the minus 6 cubic meters divided by Planck's constant times speed of light and then all that gets multiplied by the specific heat of water times the change in temperature, which is the difference between 100 degrees Celsius and 37 degrees Celsius and then add to that the latent heat of vaporization and we get 1.34 times 10 to the 23 photons. Part (b) is asking for the minimum power output during this flash from the laser so that will be the energy divided by time and the energy is the number of photons times Planck's constant times speed of light divided by wavelength and all that gets divided by time which we are told is 1.00 millisecond. So we have the answer to part (a) times Planck's constant times speed of light divided by the wavelength here— this is λ—and then all that gets divided by the time, which is 1.00 times 10 to the minus 3 seconds and this works out to 2.52 megawatts.