Question
(a) Calculate for a proton that has a momentum of . (b) What is its speed? Such protons form a rare component of cosmic radiation with uncertain origins.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 42 (Problems & Exercises)
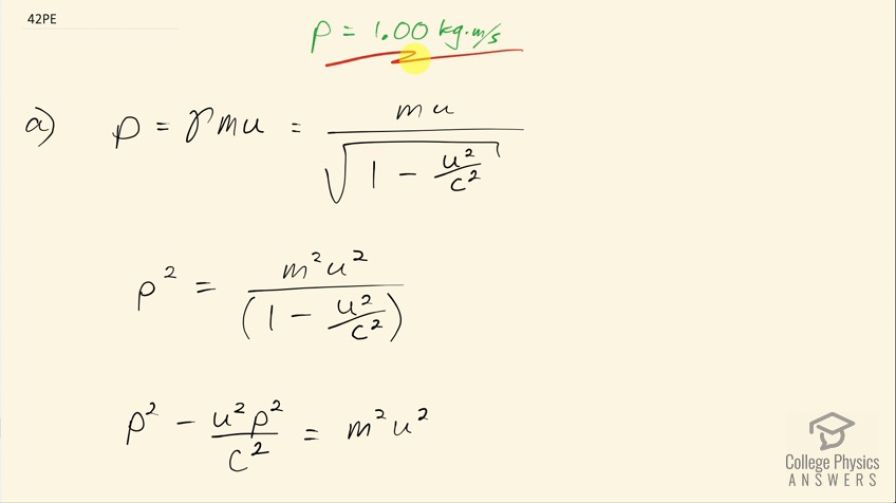
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A proton has momentum of 1.00 kilogram meters per second, which is a massive amount of momentum for a proton given the small amount of rest mass that it has. So the momentum of a proton is this Lorentz factor γ times its rest mass times its velocity, in part (a), we have to figure out what is gamma? Well if we replace it with 1 over square root of 1 minus the v squared over c squared, we can do some algebra to solve for u and then after we know what u is, then we'll replace u in this formula for γ 1 over square root 1 minus u squared over c squared. So we are going to square this side and square this side as well so we have momentum squared is rest mass squared times velocity squared over 1 minus velocity squared over c squared and then multiply both sides by 1 minus u squared over c squared and so we have momentum squared minus momentum squared times u squared over c squared equals m squaredu squared and then add u squaredp squared over c squared to both sides and then switch the sides around and we have m squaredu squared plus u squaredp squared over c squared equals p squared and we factor out u squared from both of these terms and then divide both sides by this binomial m squared plus p squared over c squared and so we have u squared is p squared over m squared plus p squared over c squared and then take the square root of both sides and so the velocity then is the momentum divided by the square root of the rest mass squared plus the momentum squared over c squared. So that's 1.00 kilogram meters per second divided by the square root of 1.67 times 10 to the minus 27 kilograms squared plus 1.00 kilogram meters per second squared over the speed of light squared and this works out to 2.998 times 10 to the 8 meters per second and so it's practically the speed of light to the precision of the calculator and the precision of the numbers that we have chosen to use here, it's almost approaching the speed of light. So γ then is 1 over the square root of 1 minus the v squared over c squared but since the velocity is essentially approaching the speed of light, this works out to 1 so γ is essentially 1.
