Question
(a) At what relative velocity is ? (b) At what relative velocity is ?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 9 (Problems & Exercises)
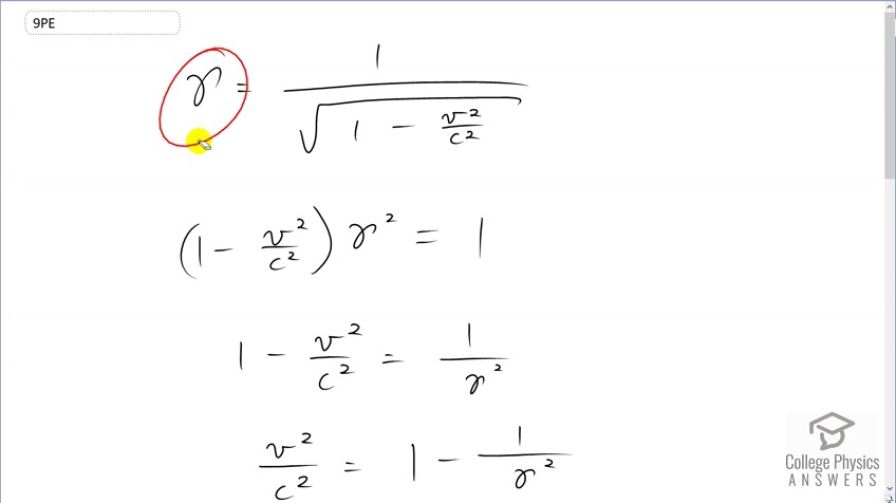
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Both parts of this question ask us to find the speed v given a certain number for gamma. So we need to do some algebra here to solve for v. Let's square both sides gets rid of the square root, it turns it into a bracket, and then multiply both sides by that bracket. So times by 1 minus v squared over c squared and it cancels on the right hand side here and then on the left side, we end up with 1 minus v squared over c squared times gamma squared. And that equals 1 on the right side. Then we'll divide both sides by gamma squared and we get 1 minus v squared over c squared equals 1 minus gamma squared. Or sorry, I didn't say that right; 1 over gamma squared, I should say. And then add v squared over c squared to both sides and then substract this 1 over gamma squared from both sides. Then switch the sides around and you end up with v squared over c squared equals 1 minus 1 over gamma squared. Then multiply both sides by c squared and it cancels on the left and ends up being a factor on the right hand side. We have c squared times 1 minus 1 over gamma squared and then take the square root of both sides to give us v is c times the square root of 1 minus 1 over gamma squared. So now we have an expression that we can use to figure out v, given a value for gamma. So in part (a), we are told that gamma is 1.5 so we have the speed of light times the square root of 1 minus 1 over 1.50 squared giving a speed of 2.23 times 10 to the 8 meters per second. And if gamma was 100, we would have to have a speed of 2.9979 times 10 to the 8 meters per second, in order to have the Lorentz factor, gamma, be 100. This has too many significant figures considering the number of significant figures that I used to do the calculation but we are gonna keep them anyway just to distinguish this number from (c) so that we don't give a misconception that v equals c, which it never can. But it's pretty darn close and it differs a little bit though, as you can see, from this fifth significant figure here.
