Question
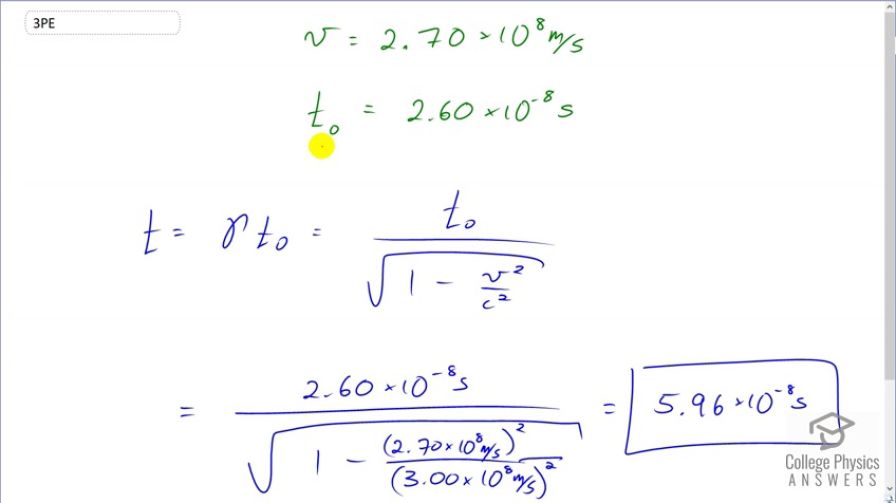
Particles called -mesons are produced by accelerator beams. If these particles travel at and live when at rest relative to an observer, how long do they live as viewed in the laboratory?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 3 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are told how long these Pi mesons live according to an observer at rest with respect to the mesons and that time is 2.6 times 10 to the minus 8 seconds. And since the observer and then the Pi meson are at rest when this time is measured, this is called proper time. And then we are told, well suppose, that the Pi meson is now moving at 2.7 times 10 to the 8 meters per second, what will the time of its lifetime be observed now and this is going to be relativistic time now because the Pi meson and the observer are not at rest with respect to each other. And so we have to multiply the proper time by this Lorentz factor—gamma— which is 1 over square root 1 minus speed squared divided by speed of light squared. And then we substitute in our proper time, 2.6 times 10 to the minus 8 seconds and we divide that by square root 1 minus the speed of the Pi meson, which is 2.7 times 10 to the 8 meters per second and we square that and divide by the speed of light squared. And this gives 5.96 times 10 to the minus 8 seconds, which is bigger than the proper time. And so because the Pi meson is moving, its lifetime has gotten bigger, or in other words, dilated. And so this is time dilation as a result of the high speed of the Pi meson.
