Question
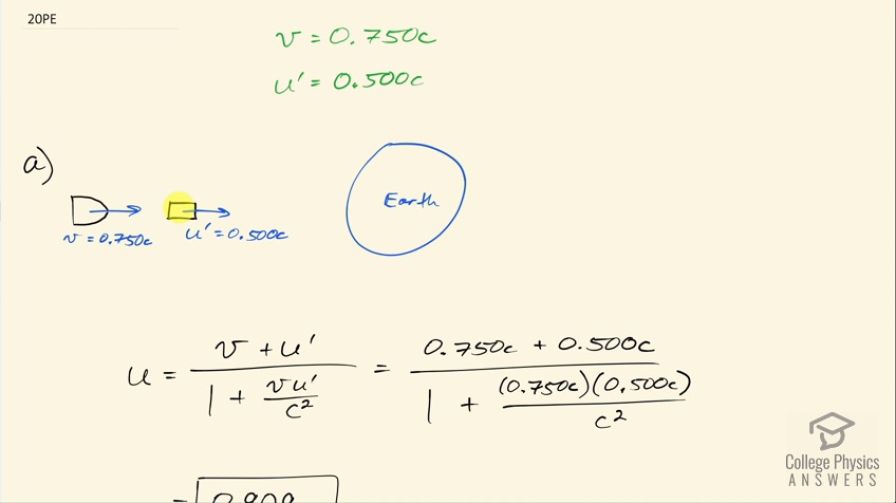
Suppose a spaceship heading straight towards the Earth
at can shoot a canister at relative to the
ship. (a) What is the velocity of the canister relative to the Earth, if it is shot directly at the Earth? (b) If it is shot directly away from the Earth?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 20 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A spaceship is heading towards Earth with a speed of 0.750c and the spaceship launches some canister towards the Earth with a speed of 0.500c as measured by a person in the spaceship and the question is what speed does a person who is on the Earth measure for this approaching canister? So the velocity of the canister is going to be the velocity of the spaceship plus the velocity of the canister according to the person on the spaceship— that's what this prime represents— u prime is the speed measured by a person who is moving at v and that's divided by 1 plus v times u prime over c squared. So that's 0.750c plus 0.500c all divided by 1 plus 0.750c times 0.500c over c squared and this is 0.909c is what it works out to. Okay! And we expected some answer that was greater than 0.750 and according to our Galilean relativity, we would have expected, you know, the Galilean relativistic velocity to be 0.750 plus 0.500 but as we know from this chapter, it doesn't quite work like that but we do expect some number greater than 0.750. Okay! There we go! Part (b) says same scenario but the canister is being propelled away from the Earth at a speed of 0.500c so that makes its velocity—u prime— negative 0.500c so that's the thing to notice here, the negative. So the velocity according to an Earth-based observer for the canister will be positive 0.750c because the spaceship is approaching the Earth and we have to the right being the positive direction and then plus a negative 0.500c—u prime— divided by 1 plus 0.750c times negative 0.500c over c squared and this works out to positive 0.400c and the positive means that this canister is approaching the Earth according to the Earth-based observer as we expected but it's approaching at the velocity of 0.400c.
