Question
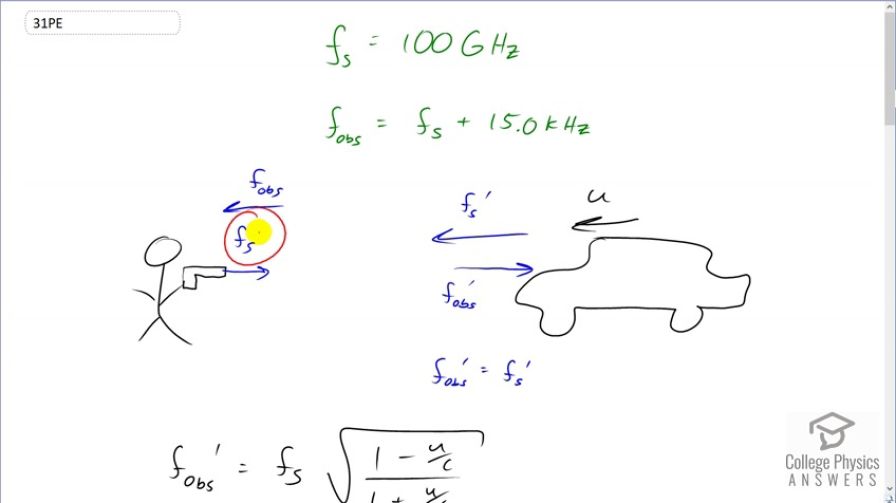
A highway patrol officer uses a device that measures the speed of vehicles by bouncing radar off them and measuring the Doppler shift. The outgoing radar has a frequency of 100 GHz and the returning echo has a frequency 15.0 kHz higher. What is the velocity of the vehicle? Note that there are two Doppler shifts in echoes. Be certain not to round off until the end of the problem, because the effect is small.
Final Answer
(negative means the vehicle is approaching the officer)
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 31 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A police officer has a radar gun that emits a frequency of 100 gigahertz; we'll call that f subscript s for the source frequency. This radar hits a moving vehicle that's approaching the police officer and the moving vehicle, an observer there, would notice a different frequency that is Doppler shifted since this observer is moving towards the stationary source and we'll call that f obs for observer. But I have written a prime here to distinguish it from this observed frequency which is the frequency observed by the police officer reflected from the car. And we are told that this frequency received by the police officer is 15 kilohertz higher than originally emitted by the police officer. Now, this frequency received by the car is reflected and we'll consider that then the source frequency for this second calculation of a relativistic Doppler shift. And this f s prime is the same as the f observed prime. This on the one hand, we'll think of it as a frequency observed by this moving observer and then on the second hand, we'll consider this car to be the source of radar that has been reflected from it and that source will have a frequency that it observed. OK. So there's two Doppler shifts to consider; the first happens because this observer is moving towards the source and the second happens because we can think of this as a moving source that reflects this frequency that has been shifted and then that's what gets observed over here by the police officer. So first step is to figure out, what is this frequency that's observed by a person in the car. So that's f observed prime. That's the originally omitted frequency, f s, times the square root of 1 minus the car's velocity over c divided by 1 plus the car's velocity over c. And then the frequency observed by the police officer is going to be whatever frequency gets emitted by the car or reflected; we'll call that f s prime times square root of 1 minus u over c over 1 plus u over c. Now this frequency observed by a person in the car is equal to the frequency that it then reflects of course. And so we can substitute this with all of this. And so we have the frequency observed in the end by the police officer is the frequency emitted originally, which is then Doppler shifted by the time it hits the car and then that reflection from the car is in turn Doppler shifted by the time it reaches the police officer. So we have this then and we have to solve for u which is the velocity of the car. So these two square roots are the same, they multiply together and so that just makes the square root disappear. So we have 1 minus u over c over 1 plus u over c times the original emitted frequency and that equals the final observed frequency by the police officer. And then we'll multiply both sides by this denominator—1 plus u over c— and distributing the f observed into the bracket here, we end up with f observed plus f observed times u over c equals source frequency minus source frequency times u over c and then we'll get rid of fractions because fractions make things a little bit messy. So we'll mutliply everything by c and we end up with c times f observed plus f observed times u equals f source times c minus f source times u. And then we'll collect the u terms on the same side so add f s u to both sides and we'll substract f observed c from both sides. And we end up with f observed times u plus source frequency times u equals source frequency times c minus f observed times c. And then we'll solve for u finally so we can factor out the u and it becomes u multiplied by f observed plus frequency of the source and then divide both sides by that bracket. And so we end up with u equals c which we can factor out from these two terms times source frequency minus observed frequency divided by the sum of the two frequencies. So the observed frequency by the police officer, we are told, is 15 kilohertz more than the frequency originally emitted and so we substitute that in for f observed on the top and bottom here. And we see that we end up with these f source disappears from the top and we end up with negative 15 kilohertz and that's a negative because this negative sign gets distributed into the two terms in the bracket there. And on the bottom, we end up with 2 times the source frequency and then plus 15 kilohertz. So the velocity of the car is speed of light, c, which is 2.998 times 10 to the 8 meters per second times negative 15 times 10 to the 3 hertz where I have written kilohertz as times 10 to the 3 hertz divided by 2 times 100 gigahertz where times 10 to the 9 is the prefix 'giga' and then plus 15 times 10 to the 3 hertz. And this works out to negative 22.5 meters per second and the negative sign means that this this object is approaching the observer.
