Question
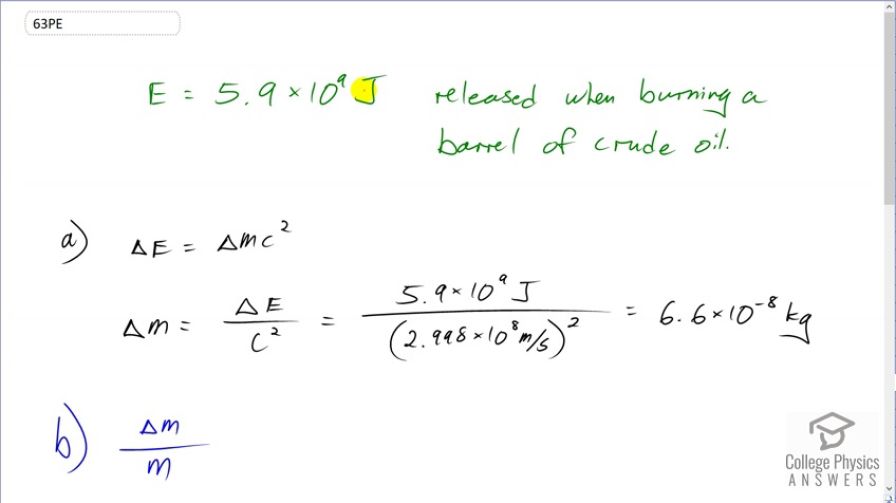
(a) Using data from Table 7.1, find the mass destroyed when the energy in a barrel of crude oil is released. (b) Given these barrels contain 200 liters and assuming the density of crude oil is , what is the ratio of mass destroyed to original mass, ?
Final Answer
- $4.4 \times 10^{-10}. This small ratio is why mass changes are not noticed after chemical reactions.
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 63 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. According to table 7.1, the energy released when burning a barrel of crude oil is 5.9 times 10 to the 9 joules. And we are going to figure out, how much mass is converted into energy in order to release this much energy? So the amount of energy released is the change in mass times c squared. And so we'll divide both sides by c squared and get the change in mass. It'll be 5.9 times 10 to the 9 joules divided by the speed of light squared, which is 6.6 times 10 to the minus 8 kilograms, which is 66 nanograms, a very small amount. And then in part (b), we want to figure out what ratio of the original mass of oil does this mass loss represent? So we are told that the density of oil is 750 kilograms per cubic meter and that a barrel consists of 200 liters. So we want to figure out what is we know what ΔM is, that's what we figured out in part (a), we need to figure out the original mass of the barrel of oil. So density is mass divided by volume and we can solve for m by multiplying both sides by v so m is density times v and then we can substitute this in for m in this ratio. So I have ΔM over ρV and that's gonna be 6.564 times 10 to the minus 8 kilograms, our answer from part (a), the amount of mass that was converted into energy and divided by mass of the entire barrel of oil. So that's 750 kilograms per cubic meter then we'll multiply by 200 liters but need to convert this unit of liters into cubic meters by multiplying by 1 cubic meter for every 1000 liters and so then the cubic meters will cancel leaving us with kilograms in the bottom. This works out to 4.4 times 10 to the minus 10 and that is a very small, small number and that small ratio is why mass changes are not noticed after chemical reactions.
