Question
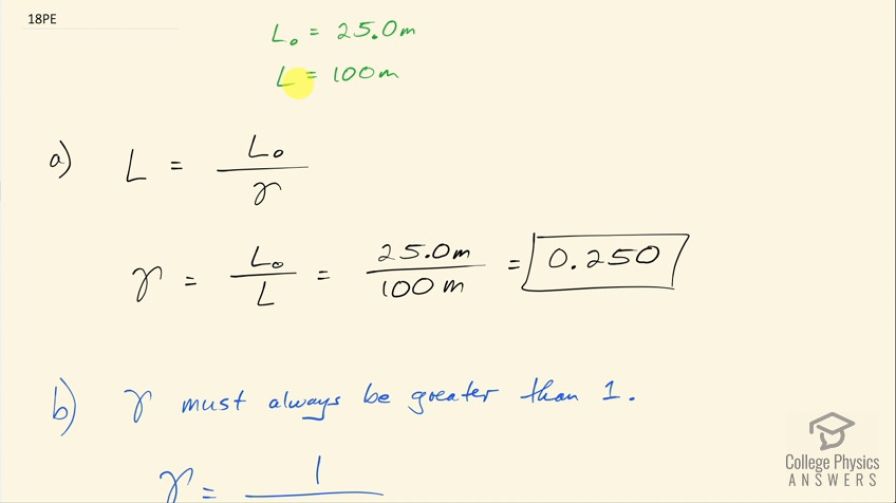
(a) Find the value of for the following situation. An
astronaut measures the length of her spaceship to be 25.0 m, while an Earth-bound observer measures it to be 100 m. (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?
Final Answer
- must always be greater than 1.
- It's unreasonable to think the Earth based observer would measure a greater length.
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 18 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. What is the relative speed when the Lorentz factor γ is 1.03? So γ is 1 over the square root of 1 minus the relative speed squared over c squared and we can multiply both sides by 1 minus v squared over c squared and divide both sides by γ and we get square root 1 minus v squared over c squared is 1 over γ and then we can square both sides and get 1 minus v squared over c squared is 1 over γ squared and then add v squared over c squared to both sides and subtract 1 over γ squared from both sides and we get v squared over c squared is 1 minus 1 over γ squared then multiply both sides by c squared and you get v squared is c squared times 1 minus 1 over γ squared then square root both sides and finally we have a formula for the speed v in terms of speed of light and γ. So we have speed of light times square root 1 minus 1 over γ squared. So that's 2.998 times 10 to the 8 meters per second times square root 1 minus 1 over 1.03 squared and that's 7.183 times 10 to the 7 meters per second. So at speeds greater than this, you will have a Lorentz factor that is creating a relativistic effect that exceeds 3 percent.