OpenStax College Physics for AP® Courses, Chapter 28, Problem 5 (Problems & Exercises)

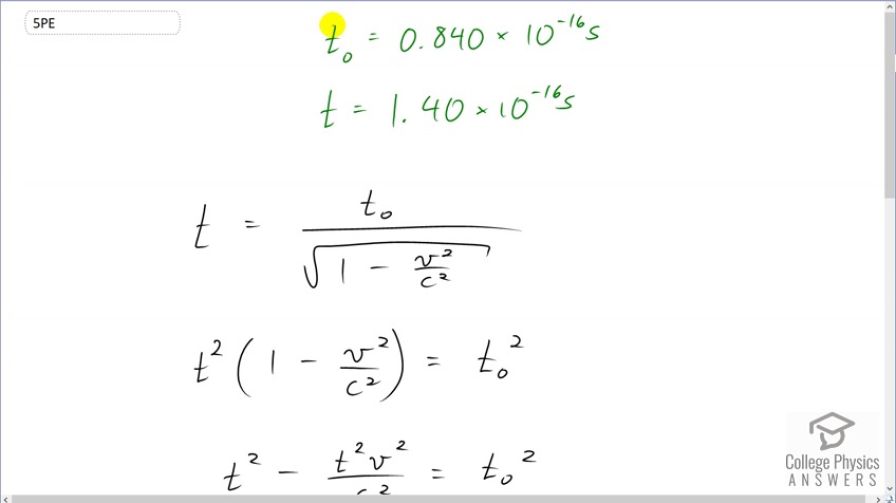
This is College Physics Answers with Shaun Dychko. The lifetime of this neutral Pi meson is measured in two different circumstances; in the one circumstance, it is at rest with respect to the observer and that time is gonna therefore be called proper time and it's 0.840 times 10 to the minus 16 seconds. And then when the Pi meson is moving, it has a different time for its lifetime, which is 1.40 times 10 to the minus 16 seconds. And we know that the relativistic time measured when the Pi meson is moving, is going to equal the Lorentz factor—gamma— multiplied by the proper time, proper meaning that it's measured at rest with respect to the Pi meson. And so gamma is 1 over square root 1 minus the speed of the Pi meson squared divided by speed of light squared and we have to solve this for v, in order to figure out how fast the Pi meson is moving in order to have this relativistic time for its lifetime. So, first thing we'll do is square both sides and also multiply both sides by this denominator and we end up with this second line here. So we have proper time squared equals relativistic time squared times 1 minus v squared over c squared and the square root sign disappeared because we are squaring the square root. And so everything got squared here and then we multiply both sides by this denominator to bring it into the numerator on the other side. Then we'll distribute the t squared into the brackets and so we have t squared multiplied by 1 is t squared minus t squared v squared over c squared equals proper time squared; it kinda sounds like Dr. Seuss, t squared v squared over c squared. Anyway, we are going to bring this to the right hand side by adding it to both sides and then take this to the left hand side by subtracting it from both sides and then we end up with, after switching the sides around, t squared v squared over c squared equals t squared minus t naught squared. Then isolate v squared by multiplying both sides by c squared over t squared and you end up with v squared on the left equals c squared over t squared times t squared minus t naught squared. And then take the square root of both sides. So on this right hand side, we have this factor multiplied by this bracket and we can take the square root of each of them separately and then multiply the results together. So this c squared over t squared, we'll write as c over t and then the bracket, the square root of it, is we can't simplify that so we just have to write square root of t squared minus t naught squared. And then we plug in numbers now that we have a formula for the speed of the Pi meson in terms of its relativistic time and proper time. So we have 2.998 times 10 to the 8 meters per second—speed of light— divided by the time measured when it's moving—1.40 times 10 to the minus 16 seconds— times the square root of that relativistic time squared minus the proper time squared giving 2.40 times 10 to the 8 meters per second must be the speed of the neutral Pi meson.