Question
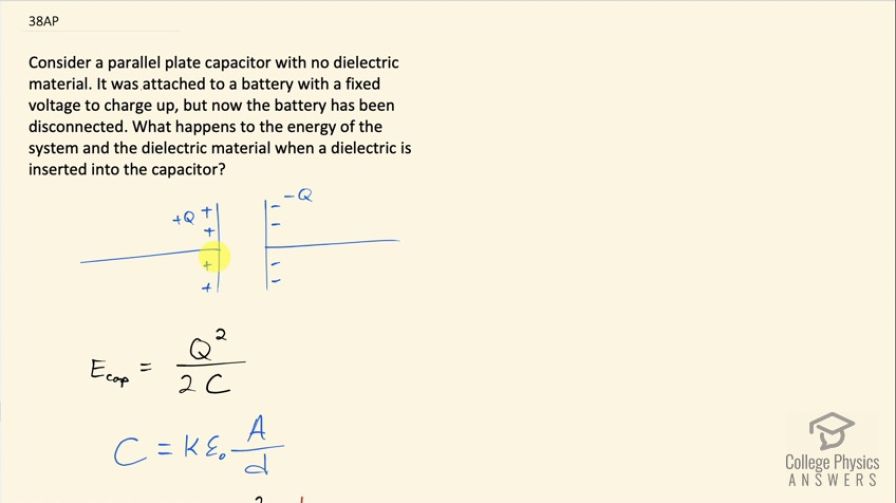
Consider a parallel plate capacitor with no dielectric material. It was attached to a battery with a fixed voltage to charge up, but now the battery has been disconnected. What happens to the energy of the system and the dielectric material when a dielectric is inserted into the capacitor?
Final Answer
The energy stored by the capacitor decreases when the dielectric is inserted. the capacitor does work on the dielectric to pull it into place.
Solution video
OpenStax College Physics for AP® Courses, Chapter 19, Problem 38 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. A parallel plate capacitor is charged up by a battery but then the battery is removed from the circuit and so we are just left with a capacitor that is charged so it has positive charge on one plate and negative charge on the other and the energy stored in this capacitor is the amount of charge squared divided by 2 times its capacitance and we want to know what happens when a dielectric is inserted into this space between the plates what happens to the energy stored in the capacitor? So we have this energy now in terms of the dielectric constant— this is a property of the material that's put in here— and it's going to be a number greater than or equal to 1 so I mention that down here. And so this means the energy stored in the capacitor is inversely proportional to this dielectric constant but I am getting ahead of myself here... let's make the substitution in place of C here. So we are going to divide by C, which means multiply by this flipped over and so we have d over Κε naught times area and the amount of charge doesn't change when the dielectric is put in here— the dielectric isn't going to move charge between these plates at all— and so this Q remains constant the separation between the plates is constant the plate area of course is constant this is the permittivity of free space that's a constant so the only thing that can change here is this dielectric constant due to the material being introduced. So this means we can say that the energy stored by the capacitor is proportional to 1 over the dielectric constant. and that means the energy stored by the capacitor decreases when the dielectric is inserted because since this dielectric constant is always a number greater than or equal to 1, taking the reciprocal of a number like that is a number less than 1 so this energy stored in a capacitor will end up being less than it was before and this means the capacitor actually does some work on the dielectric to pull it into place.