Question
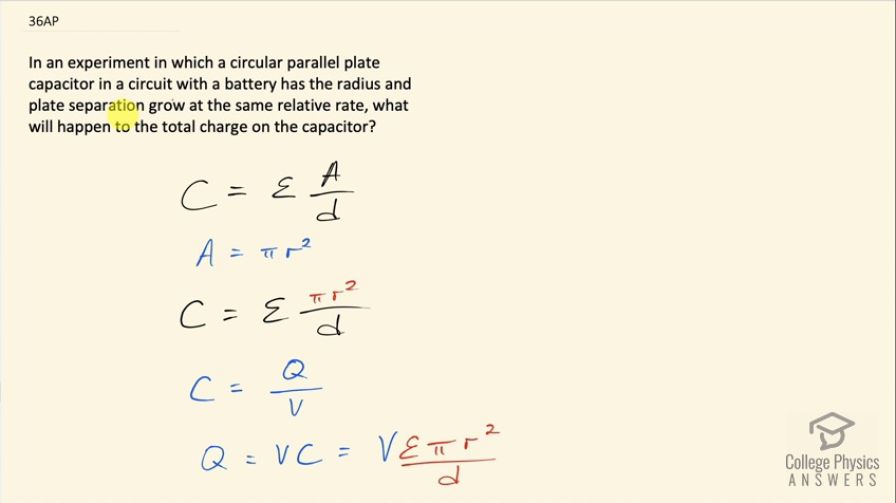
In an experiment in which a circular parallel plate capacitor in a circuit with a battery has the radius and plate separation grow at the same relative rate, what will happen to the total charge on the capacitor?
Final Answer
The charge on the capacitor will grow by the same factor as the radius and separation.
Solution video
OpenStax College Physics for AP® Courses, Chapter 19, Problem 36 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. A capacitor with circular plates is connected to a battery and the radius and the plate separation of the capacitor grow at the same rate so they are growing by the same factor... they will be multiplied by the same factor... what will happen to the total charge in the capacitor? So the capacitance is the permittivity of whatever material is between the plates times by the area of each plate divided by the separation between the plates and the area is π times the radius of the plate squared since we are told it's circular and so then the capacitance then is this permittivity times πr squared over d. We can also relate capacitance to charge because the question is what is the total charge and so that's what Q is in this formula so capacitance is the amount of charge per volt and we can multiply both sides by the voltage across the capacitor and that gives us the amount of charge stored on it it's the voltage times the capacitance. So that's voltage times permittivity times πr squared over separation between the plates. So let us suppose that the growth factor by which the radius and the separation change is going to be x and since both the radius and separation change by the same factor, we'll say that the radius in the second case is x times the radius in the first case and the separation in the second case is the same factor x times the separation in the first case. So Q 1 then is Vεπr 1 squared over d 1 and the charge in the second case is Vεπr 2 squared over d 2 and the v does not get a subscript because the voltage is determined by the battery in the circuit... we are told that the capacitor's connected to a battery and the battery has a constant defined voltage and we can replace r 2 with xr 1 and replace < i>d 2 with xd 1 and then this becomes x squaredr 1 squared divided by x times d 1 and x squared divided by x is x and so we are left with Vεπr 1 squared over d 1 times x and this whole thing is Q 1 so we make that substitution here and that means Q 2 then is this factor x times Q 1. So the charge in the capacitor will grow by the same factor as the radius and the separation because the charge in the second case will be that same factor by which the radius and separation were growing multiplied by Q 1.