OpenStax College Physics for AP® Courses, Chapter 19, Problem 14 (Test Prep for AP® Courses)

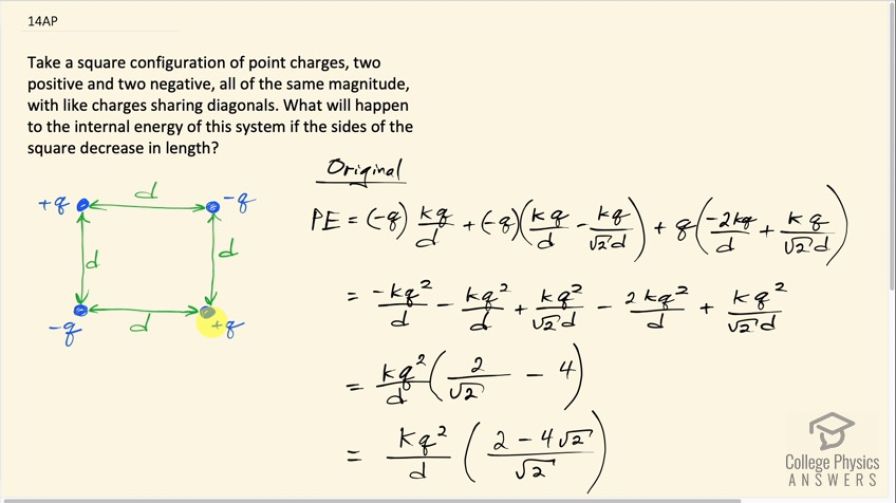
This is College Physics Answers with Shaun Dychko. Charges are arranged on the corners of a square and the diagonals are the same sign charge so there's a positive here say and then on the diagonal from that there's another positive and then this is negative and this one's negative and the side length I have labeled d and the question is how does the internal energy of the system change as the side length decreases? So we'll need to create an expression for the potential energy of the system— potential energy and internal energy are the same thing here— so to figure out the potential energy of this configuration, we'll imagine that suppose this charge is the first one to appear on the scene in which case there's no work needed to put it there because there's no other charges existing yet and then we introduce this charge and the potential energy of this charge will be its charge negative q multiplied by the potential of this position due to the single plus q charge and that is kq over the distance d. Then we introduce this negative charge to a system consisting of this positive and this negative charge and this one has not appeared on the scene yet by the way so we have this negative q multiplied by the potential of this position due to this charge at the top left and this charge at the top right and so it's negative q times kq over d— kq over d is the potential due to this positive charge q at this position here— and then we have a negative potential due to this negative q charge and that's gonna be minus kq over the distance from this negative q charge to this negative q charge so this distance here is the hypotenuse of this right triangle here with side lengths d and the length of the hypotenuse will be square root 2 times d and so we are dividing kq by this hypotenuse distance root 2 times d. Okay! And then lastly we introduce this fourth charge—positive q—into a system consisting of these three charges already and so we multiply this charge q by the potential at this position due to the other three charges two of them are a distance d away with a charge of negative q so we have negative 2 multiplied by kq over d and then add to that the potential due to this positive q charge on the diagonal and that's kq over root 2d. So multiply into the brackets and then we end up with this line here— negative kq squared over d— minus kq squared over d plus kq squared over root 2d minus 2kq squared over d plus kq squared over root 2d. So we have kq squared over d is a common factor among all these terms so we can factor that out and then we are left with negative 1 here after factoring out kq squared over d and then negative 1 here and then negative 2 here so that accounts for this minus 4 and then we have 1 over root 2 and another 1 over root 2, which is 2 over root 2 due to these two factors and giving them a common denominator makes this 2 minus 4 root 2 all over root 2 because I multiply the 4 by the number 1, which I have written as root 2 over root 2 and this works out to this line here and so we at long last have an expression for the potential energy of the whole system. And we can see that the potential energy is inversely proportional to the side length and so that means as the side length decreases, this quotient will increase or in other words, the magnitude of the potential energy will increase and I am specific about saying magnitude because you know if this whole thing is negative, which it is because 2 minus 4 root 2 is negative... as d decreases, this quotient will become more negative which strictly speaking is to say it will decrease but I wanna bring attention to the fact that its size will increase. Okay! So that's one way to say things or another way to say things is that the potential energy will become further negative as d decreases.