Question
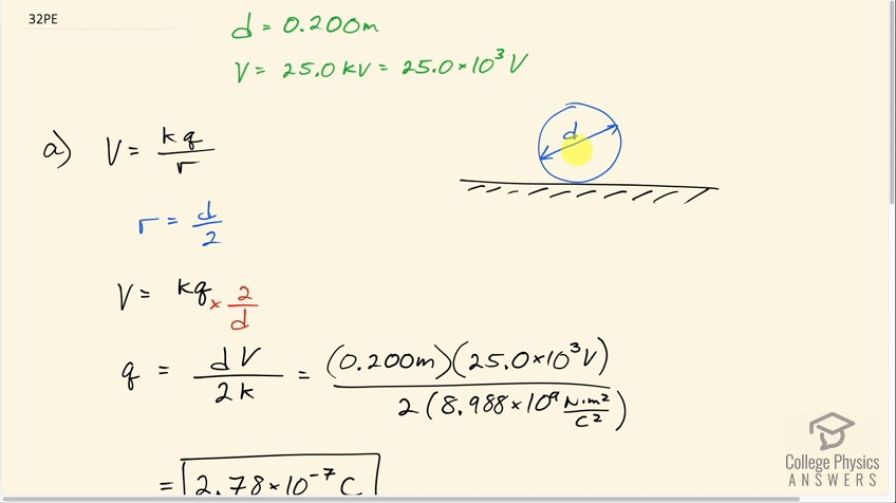
An electrostatic paint sprayer has a 0.200-m-diameter metal sphere at a potential of 25.0 kV that repels paint droplets onto a grounded object. (a) What charge is on the sphere? (b) What charge must a 0.100-mg drop of paint have to arrive at the object with a speed of 10.0 m/s?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 19, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This metal sphere is part of an electrostatic paint sprayer and is gonna paint this black surface here and the potential difference between the sphere and the surface is 25.0 kilovolts, which is 25.0 times 10 to the 3 volts. The diameter of the sphere is 0.200 meters and we can model the sphere as a single point charge at its center and the question is how much charge would be there which will equal the total charge on the whole surface? So this point charge is some distance, r, which is the radius of the sphere away from the surface and its potential is Coulomb's constant times the charge divided by that distance r. So r is half the diameter so we can divide by d over 2 or multiply by its reciprocal so instead of dividing by this fraction, I prefer to multiply by the reciprocal of a fraction. So we have kq times 2 over d and then we can solve this for q by multiplying both sides by d over 2k q equals dV over 2k. So that's 0.200 meters times 25.0 times 10 to the 3 volts divided by 2 times 8.988 times 10 to the 9 newton meters squared per coulomb squared, which is 2.78 times 10 to the minus 7 coulombs. Now in part (b), it asks what charge must a 0.100 milligram drop of paint have to arrive at the object with a certain speed of 10.0 meters per second? So the kinetic energy of a droplet of paint will be the charge on the droplet multiplied by the potential difference through which it travels, V, and so this q here is not the same as this q here... perhaps I should have used a different letter but this is the charge on the drop of paint. This kinetic energy is also one-half times mass times its speed squared and so we'll make that substitution and we show that here—one-half mv squared equals q times V— and we'll solve for q now by dividing both sides by the voltage. So the charge on a drop of paint is the paint's mass times its speed squared divided by 2 times the voltage through which it travels. So that's 0.100 milligrams times 1 gram for every 1000 milligrams times 1 kilogram for every 1000 grams— giving us kilograms here— times 10.0 meters per second squared divided by 2 times 25.0 times 10 to the 3 volts and that is 2.00 times 10 to the minus 10 coulombs of charge needed on the 0.100 milligram drop of paint.
