OpenStax College Physics for AP® Courses, Chapter 32, Problem 42 (Problems & Exercises)

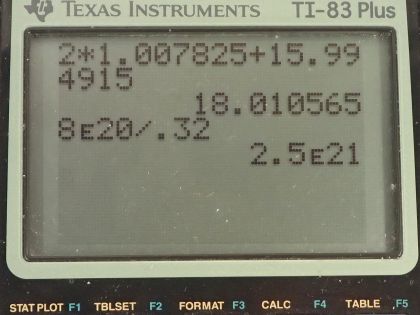
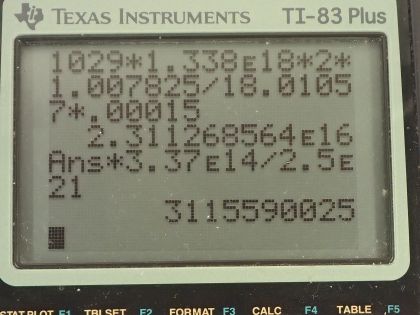
This is College Physics Answers with Shaun Dychko. We are going to estimate for how many years would the fusion of deuterium in the worlds oceans provide for all the energy consumption of the entire planet? So we are going to assume energy consumption is 10 times that of the United States so that's 10 times 8 times 10 to the 19 joules per year so that's 8 times 10 to the 20 joules of energy consumed per year. The efficiency of producing energy is 0.32 and we are told that 0.015 percent of all the hydrogen is of the isotope deuterium and the energy produced by deuterium fusion is 3.37 times 10 to the 14 joules per kilogram of deuterium. I looked up the volume of worlds oceans and it's about 1.338 times 10 to the 9 cubic kilometers and then converting them to meters by multiplying by 1000 meters per kilometer three times is 1.338 times 10 to the 18 cubic meters; the molar mass of hydrogen is 1.007825 grams per mol and then we looked up molar mass of oxygen and then here's the density of seawater— 1029 kilograms per cubic meter. So we are going to first calculate the molar mass of water so that's 2 times the hydrogen molar mass plus oxygen molar mass for 18.01057 grams per mol and this is important because we will need to figure out what fraction the hydrogen is of the total water molecule's mass. We will see that in a second. Efficiency is the energy consumed divided by the total energy produced and we want to know what is the energy that needs to be produced from this deuterium so we rearrange this to solve for E p by multiplying both sides by E p divided by efficiency and we get the energy produced then is the energy consumed divided by efficiency so that's 8 times 10 to the 20 joules per year divided by 0.32 and that's 2.5 times 10 to the 21 joules per year that need to be produced. What mass of deuterium is in the ocean? Once we know that mass, I will give you a little look in the future here of what's happening... we need to know what the mass is of deuterium and then we are going to multiply that by the amount of energy produced by fusion of deuterium for every kilogram of deuterium and then multiply that by the amount of time per energy consumed okay... or energy produced, I should say because this is how much energy we need to get out of deuterium. Okay and that's what we calculated up here. Alright! So the mass of deuterium in the oceans is the density of the oceans— 1029 kilograms of water for every cubic meter of water— and I knew that I should start with this fraction because it has units of kilograms in the numerator and we want to have kilograms in our answer. Then we multiply this by the number of cubic meters of water so that the cubic meters cancel that's 1.338 times 10 to the 18 cubic meters and then the water things cancel too— water like isn't a unit but you can still think of it as that anyway... at least I do and I think it helps me figure out where to write things. Then we are going to multiply this by what fraction of this mass of water is in the form of hydrogen? And that will be, you know, 2 times the hydrogen's molar mass divided by the molar mass of the water overall and so we have water canceling here and we have grams per mol canceling and we have kilograms now of hydrogen. Then we multiply that by how much deuterium we have for every hydrogen atom so that's 0.00015 deuterium for every hydrogen and so now we have kilograms of deuterium and that is 2.3113 times 10 to 16 kilograms of deuterium. So the time that this deuterium fusion will produce enough energy for is 1 year for every 2.5 times 10 to the 21 joules produced times 3.37 times 10 to the 14 joules per kilogram of deuterium that's fused and then multiply by 2.3113 times 10 to the 16 kilograms of deuterium and we are left with an answer in years and that's 3 times 10 to the 9 years— that's 3 billion years. This is about 3 times longer than humans are expected to exist on Earth because the Sun's fusion chain of events is going to change such that in a billion years, there's going to be about a 10 percent increase in the intensity of radiation from the Sun which will cause the oceans to evaporate and life won't exist once that happens because then there will be a massive greenhouse effect as a result of all this water vapor in the air and furthermore, the Sun's cosmic rays will split apart oxygen molecules into... or water molecules into hydrogen and oxygen, which will then escape into space. So humans are expected to live on Earth for about another billion years and this is 3 times as long as that.