Question
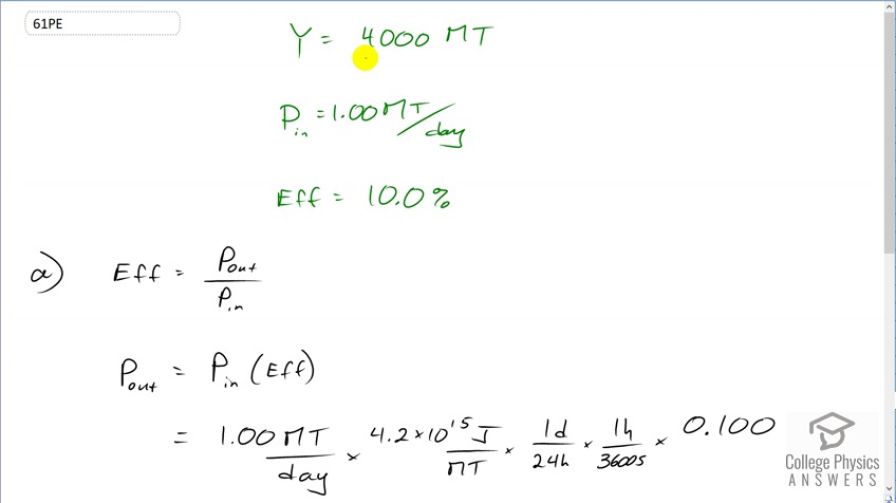
One scheme to put nuclear weapons to nonmilitary use is to explode them underground in a geologically stable region and extract the geothermal energy for electricity production. There was a total yield of about 4,000 MT in the combined arsenals in 2006. If 1.00 MT per day could be converted to electricity with an efficiency of 10.0%:
(a) What would the average electrical power output be? (b) How many years would the arsenal last at this rate?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 32, Problem 61 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The combined yield of all the world's nuclear weapons in year 2006 was 4000 megatons and if those weapons were put underground and exploded to make heat that could then be used to create electricity, what would the rate of electricity output be assuming an efficiency of 10 percent conversion of that thermal energy into electrical energy? And we'll explode 1 megaton worth of nuclear weapons per day suppose. So efficiency is the power output divided by the power input and the output is the electrical output and the input is the geothermal energy produced by the nuclear weapons. So we multiply both sides by input and the power output then is P in times efficiency and that is 1 megaton per day times 4.2 times 10 to the 15 joules per megaton and then multiply that by these units here to turn it into mks units because days is not an mks unit so multiply by 1 day for every 24 hours and then multiply by 1 hour for every 3600 seconds and now we have joules per second which is units of watts and then multiplied by the efficiency of 0.1 that's 10 percent written as a decimal and we have 4.86 gigawatts of electrical energy produced per day. And how long would this arsenal last given this rate of exploding them? So the P in is the rate of geothermal energy production from the explosions and that is the energy in divided by time and we'll solve for t by multiplying both sides by t over P in. And we have the time then is the energy divided by the power so that's 4000 megatons total energy of all the nuclear weapons divided by 1 megaton exploded per day and this gives units of days and then we multiply that by 1 year for every 365.25 day and we end up with 11.0 years.
