OpenStax College Physics for AP® Courses, Chapter 32, Problem 28 (Problems & Exercises)

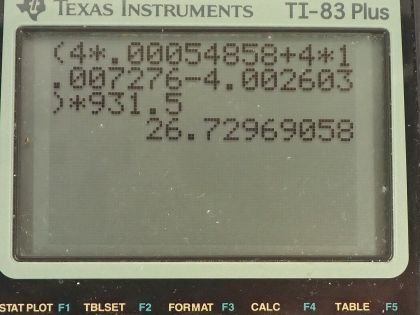
This is College Physics Answers with Shaun Dychko. We are going to calculate the total energy released in proton-proton fusion cycle. So we start with 2 protons that bind together to make a single deuterium nuclide, which has a proton and a neutron in it and this also creates a positron and an electron-neutrino and this happens twice in order to create 2 deuterium nuclides which will be needed to create 2 helium-3 nuclides that then go together to make the final helium-4 nuclide plus two protons. So I have also added an electron on the left side here because this positron will annihilate with some electron that it will find somewhere nearby and so that's going to be the mass of this electron here that this positron annihilates with will be part of the energy that is released in this reaction. So we have this happening twice and then the second line happening twice and then the third line happens once for every pair of the first two lines. So in total we have on the left side, the things that are being consumed in the reaction from the environment are two protons here and two protons here and two protons in this second line here. This one—this deuterium nuclide— I don't consider as being consumed from the environment because it's a product of this first step but this proton here in this second line is being taken from the environment and this second line happens twice and so that's a total of 2 protons from this second line and then two pairs from the first line for a total of 6 protons being consumed in this proton-proton chain. There will also be two electrons consumed from the environment as well so this is all the reactants so to speak, these are all the things that are consumed, used up in the reaction. And then the products are 2 gamma-ray photons that are produced in the second line here plus an additional 4 gamma-ray photons— 2 from each electron-positron annihilation— that occurs in this first step here. So when one electron and one positron annihilate together, they each create a photon going off in opposite directions so there's a pair of photons for every positron-electron pair and there are two positron-electron pairs for a total of 4 protons and then add to that 2 more from this second line here so that's a total of 6 photons. Okay! And then we have two electron-neutrinos... neither the photon nor the neutrino have any mass and so we won't really be able to do calculations for the energy released using the photons or the electron-neutrinos but we'll just have to assume that their energy is fairly negligible compared to the mass energy of the helium nuclide. So... we had two protons produced in this third line here and we used up 6 protons but since we used up 6 but got 2 in return that's a total of 4 that are used up and so the left side here is 4 protons have been consumed plus 2 electrons to produce a single helium-4 nuclide plus some massless particles here. So the energy released then is the mass difference between the left and the right hand side here times c squared so that's 2 electron masses plus 4 proton masses; I chose not to use the atomic mass of hydrogen instead I am writing in the mass of a bare proton here times 4. Now because I have used just a proton mass here when I look at helium, I don't know what the mass of the helium nuclide is but I can look up the atomic mass of helium-4 and so it's because an atomic mass of helium-4, we have to subtract away the two electron masses that are included in the atomic mass for helium because the word atomic includes electrons along with the nucleus and so atomic mass includes the electron masses that would normally be around helium which has 2 protons and therefore 2 electrons so we subtract those 2 electrons away. And so this minus distributed into the brackets makes a positive 2m e, which combines with this 2 times mass of an electron for a total of 4. So we have 4 times the mass of an electron plus 4 times the mass of a proton minus the atomic mass of helium-4 and all that gets multiplied by c squared. So that's 4 times 0.00054858 atomic mass units for an electron plus 4 times 1.007276 atomic mass units for a proton minus 4.002603 atomic mass units for the helium-4 atom and all that gets converted into megaelectron volts per c squared for every atomic mass unit by multiplying by 931.5 and then multiply by c squared and we are left with an answer in megaelectron volts and that is 26.73 megaelectron volts energy released.