Question
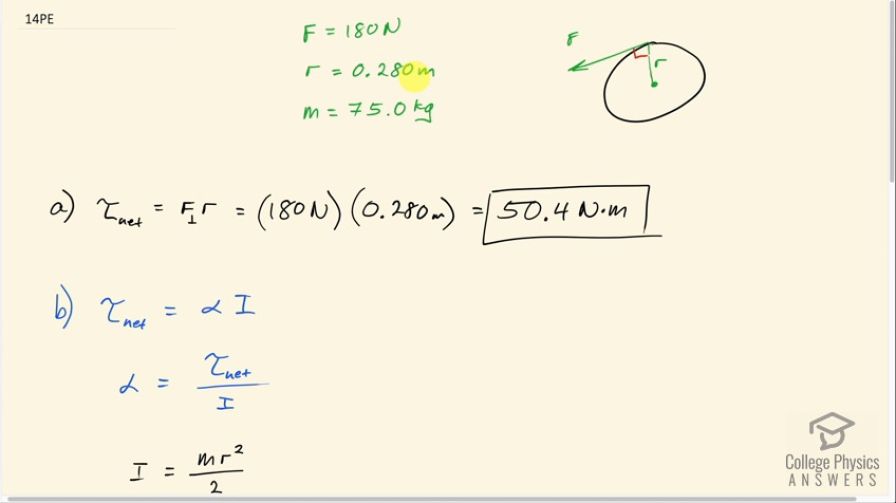
Suppose you exert a force of 180 N tangential to a 0.280-m-radius 75.0-kg grindstone (a solid disk). (a)What torque is exerted? (b) What is the angular acceleration assuming negligible opposing friction? (c) What is the angular acceleration if there is an opposing frictional force of 20.0 N exerted 1.50 cm from the axis?
Final Answer
Solution video
OpenStax College Physics, Chapter 10, Problem 14 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A 180 newtons force is exerted tangentally on a grindstone of radius 0.280 meters and the grindstone has a mass of 75.0 kilograms. Now since the force is exerted tangentally that means it is perpendicular to this radius and so this radius is the lever arm for the force and so the force and lever arm are perpendicular here so to find the torque, we just multiply them directly. So that's 180 newtons times 0.280 meters which is 50.4 newton meters of torque. In part (b), we are asked what the angular acceleration is assuming there's no friction. So we can also write an expression for net torque as angular acceleration multiplied by moment of inertia and we can divide both sides by I and solve for α. Now this moment of inertia we can find by consulting our list of formulas here: we have this formula for a solid cylinder or in this case, a really thin cylinder which is a disc in other words and it has a moment of inertia of its mass times its radius squared divided by 2 so that's where this comes from. So we are going to divide τ by this fraction but I don't like dividing fractions by fractions so instead I'm going to multiply by the reciprocal of this fraction. So I'm multiplying by 2 over mr squared instead of dividing by mr squared over 2. Okay! So the angular acceleration then is 2 times 50.4 newtons meters divided by 75.0 kilograms times 0.280 meters squared which is 17.1 radians per second per second. In part (c), we are told that there's a friction force now acting at a certain distance from the center and so we want to find the angular acceleration now and it's going to be this applied torque minus the torque due to the friction. And so we have 180 newtons times 0.280 meters minus 20 newtons of friction multiplied by its lever arm of 1.50 centimeters converted into meters and we end up with 50.1 newton-meters is the net torque. So that's the numerator then here for this... that's what we'll substitute for τ net in our angular acceleration formula and this is coming from this here after substituting for the moment of inertia. Okay! So we have 2 times 50.1 newton-meters divided by 75.0 times 0.280 squared and that's 17.0 radians per second squared.

