Question
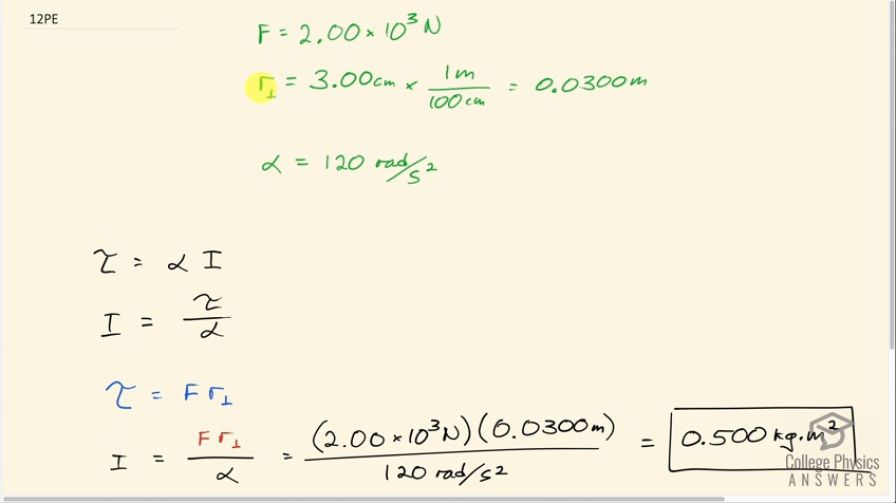
The triceps muscle in the back of the upper arm extends the forearm. This muscle in a professional boxer exerts a force of with an effective perpendicular lever arm of 3.00 cm, producing an angular acceleration of the forearm of . What is the moment of inertia of the boxer’s forearm?
Final Answer
Solution video
OpenStax College Physics, Chapter 10, Problem 12 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A boxer's forearm exerts a force of 2.00 times 10 to the 3 newtons after being accelerated by the tricep muscle that operates with a perpendicular lever arm of 3.00 centimeters which we convert into meters by multiplying by 1 meter for every 100 centimeters and this tricep muscle accelerates the forearm at 120 radians per second squared and the question is, what is the moment of inertia of the forearm? So we know that the net torque on the forearm—that's net torque— equals the angular acceleration times the moment of inertia so you can divide both sides by this α to solve for I. So moment of inertia is the net torque divided by angular acceleration and the torque is force times the perpendicular lever arm so we make a substitution F r in place of τ here and we get then that the moment of inertia is 2.00 times 10 to the 3 newtons of force times 0.0300 meters—lever arm— divided by 120 radians per second squared which is 0.500 kilogram meters squared.
